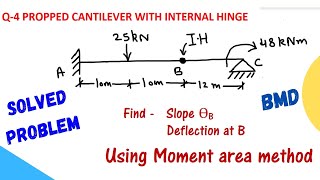
SIMPLY SUPPORTED BEAM WITH OVERHANG - SLOPE AND DEFLECTION USING MOMENT AREA METHOD | SOLVED PROBLEM

#CivilSAC
This shows how to find slope and deflection in a simply supported beam with an overhang using the moment area method. The is done in a very simple way to understand and learn the procedure easily.
Link to related videos-
SLOPE AND DEFLECTION OF SIMPLE SUPPORTED BEAM WITH MOMENT USING MOMENT AREA METHOD || SOLVED PROBLEM
• SLOPE AND DEFLECTION O...
SLOPE AND DEFLECTION OF CANTILEVER BEAM WITH POINT LOAD BY MOMENT AREA METHOD || SOLVED PROBLEM
• SLOPE AND DEFLECTION O...
SUBSCRIBE TO OUR CHANNEL TO GET LATEST VIDEO UPDATES.

[@CivilSAC]

Subscribe to my youtube channel for more tutorials.

[@ChrisPhord]

I tried using the vertical intercept from A to the tangent at B to calculate for the slope at B I had a different answer

[@saqlainmustafa7443]

Masha Allah Bhai

[@ggez7156]

Can you solve for the maximum deflection for this?

[@ChrisPhord]

Why didn’t you use tA/B for the slope at B?

[@samkeloinnocent8316]

thank you so much

[@steveharvey4329]

0A /B here geometrically will be equal to 0A + 0B...and the answer will be -208.33...i found 0B by tA/B divided by 5....for 0A getting same value of yours but applying exactly same method to 0B , where i am getting 208.33 /EI...correct me if iam doing anything wrong

[@aymanal-ahdl1694]

Yes like my solution

[@saikatdas3296]

Whole load 50kn,then how can be reaction force 75 kn😮,I think it should be 25 kn

[@CivilSAC]

The net reaction is 50 kN. Please understand that one reaction is taken upward and the other downwards. If you add them with appropriate signs, it will be 50 kN. I hope this clears your doubts.