
- 229
- 257 961
owl3
United States
เข้าร่วมเมื่อ 31 ต.ค. 2023
Welcome! This is a math channel dedicated to mini courses, playlists and RANDOM stuff from various math subjects. Focus will be Calculus, Algebra, Number Theory and more.
Great shortcut for simplifying integrals!
Website:
owlsmath.neocities.org
Check out my other channel OWLS MATH!
th-cam.com/channels/artWliFdki6px-57oVh6VA.html
Check out my other channel OWLS SCHOOL OF MATH!
th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html
#math
#integrals
owlsmath.neocities.org
Check out my other channel OWLS MATH!
th-cam.com/channels/artWliFdki6px-57oVh6VA.html
Check out my other channel OWLS SCHOOL OF MATH!
th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html
#math
#integrals
มุมมอง: 44
วีดีโอ
This trick adds 5 minutes but it's still NICE!
มุมมอง 4494 ชั่วโมงที่ผ่านมา
Laplace Transforms playlist: th-cam.com/play/PLOvxeHw2nLaySIKdV-QTjiGHHkOx0M_2-.html Practice problems: owlsmath.neocities.org/Laplace 101/laplace owlsmath.neocities.org/Inverse Laplace 101/laplace owlsmath.neocities.org/Laplace Cheat Sheet/laplace cheat sheet Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other...
Complexify the integral!
มุมมอง 61712 ชั่วโมงที่ผ่านมา
Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html Website: owlsmath.neocities.org #math #integrals #integrationtechniques #mitintegrationbee
It all went wrong when I got tan(pi/12)! (long method)
มุมมอง 59519 ชั่วโมงที่ผ่านมา
Weierstrass Substitution Intro video: th-cam.com/video/0D3VLR8Fg6g/w-d-xo.html&ab_channel=OwlsSchoolofMath Intro video: th-cam.com/video/quMwAUJzLTY/w-d-xo.html&ab_channel=OwlsMath Example problem: th-cam.com/video/rZkcobgdWFM/w-d-xo.html&ab_channel=OwlsSchoolofMath Quiz: owlsmath.neocities.org/Weierstrass Substitution/integral Formulas: owlsmath.neocities.org/Trig Identities and Formulas/trig ...
MAX Integration power
มุมมอง 881วันที่ผ่านมา
Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html Website: owlsmath.neocities.org #math #integrals #integrationtechniques #mitintegrationbee
Berkeley 2024 Calculus #5
มุมมอง 1.1Kวันที่ผ่านมา
Practice problems: owlsmath.neocities.org/Limits Quiz 2/limit owlsmath.neocities.org/Limits Quiz/limits Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html
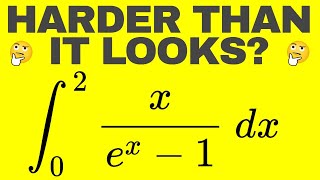
Harder than it looks? (alternative method)
มุมมอง 92314 วันที่ผ่านมา
Dilogarithm playlist: th-cam.com/play/PLOvxeHw2nLayk5tB1Lav9RDddMDOZIDBM.html Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html #math #integragls #dilogarithm
I wasn't expecting Frullani's integral here!
มุมมอง 48114 วันที่ผ่านมา
Thanks Samir! Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html Integral practice: owlsmath.neocities.org/integrals.html Website: owlsmath.neocities.org #math #integrals #integrationtechniques
UK Integration Bee 2024 #9
มุมมอง 62921 วันที่ผ่านมา
Laplace Transforms playlist: th-cam.com/play/PLOvxeHw2nLaySIKdV-QTjiGHHkOx0M_2-.html Practice problems: owlsmath.neocities.org/Laplace 101/laplace owlsmath.neocities.org/Inverse Laplace 101/laplace owlsmath.neocities.org/Laplace Cheat Sheet/laplace cheat sheet Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th...
How can I use a Laplace Transform if the bound is ln(2)???
มุมมอง 68921 วันที่ผ่านมา
Laplace Transforms playlist: th-cam.com/play/PLOvxeHw2nLaySIKdV-QTjiGHHkOx0M_2-.html Practice problems: owlsmath.neocities.org/Laplace 101/laplace owlsmath.neocities.org/Inverse Laplace 101/laplace owlsmath.neocities.org/Laplace Cheat Sheet/laplace cheat sheet Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other...
Can we generalize the Bernoulli Integral?
มุมมอง 1K21 วันที่ผ่านมา
Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html Website: owlsmath.neocities.org #math #integrals #integrationtechniques
Dilogarithm Reflection Formula
มุมมอง 47928 วันที่ผ่านมา
Dilogarithm playlist: th-cam.com/play/PLOvxeHw2nLayk5tB1Lav9RDddMDOZIDBM.html Series Cheat Sheet: owlsmath.neocities.org/Series Cheat Sheet/Series cheat sheet Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html #math #series #dilogarithm
MIT 2023 Quarterfinals #2-1
มุมมอง 833หลายเดือนก่อน
Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html Website: owlsmath.neocities.org #math #integrals #integrationtechniques #mitintegrationbee
Can you do it in 4 minutes???
มุมมอง 1.2Kหลายเดือนก่อน
A couple of shortcuts that might help: 1. reduce the integrals of something of the form sqrt(u^2 a^2) du and use "the formula" for that. Not sure if it helps since that formula is a mess but its worth a try. 2. Don't use the principle of even functions like I did. If you leave it as it is it will clean up the ugly bounds for you and then you can use even functions at the very last second. It on...
Calculate Dilogarithm(1/2) by integral
มุมมอง 802หลายเดือนก่อน
Series Cheat Sheet: owlsmath.neocities.org/Series Cheat Sheet/Series cheat sheet Website: owlsmath.neocities.org Check out my other channel OWLS MATH! th-cam.com/channels/artWliFdki6px-57oVh6VA.html Check out my other channel OWLS SCHOOL OF MATH! th-cam.com/channels/AJaLg-yEcvhC_ggEr0Hl6g.html #math #series #dilogarithm
Yes, you know the answer but...alternative methods
มุมมอง 457หลายเดือนก่อน
Yes, you know the answer but...alternative methods
Maybe the longest possible way to do it
มุมมอง 979หลายเดือนก่อน
Maybe the longest possible way to do it
This one is NICE! using the Dilogarithm
มุมมอง 1.5Kหลายเดือนก่อน
This one is NICE! using the Dilogarithm
Can I use Geometric series when x goes to infinity?
มุมมอง 1Kหลายเดือนก่อน
Can I use Geometric series when x goes to infinity?
Can we solve an infinite polynomial for x?
มุมมอง 7Kหลายเดือนก่อน
Can we solve an infinite polynomial for x?
I still found a way to sneak a Laplace Transform into this one
มุมมอง 603หลายเดือนก่อน
I still found a way to sneak a Laplace Transform into this one
Can I use Feynman's trick on this? 🤔🤔🤔
มุมมอง 1K2 หลายเดือนก่อน
Can I use Feynman's trick on this? 🤔🤔🤔
UK 2024 #8 Alternative method / Trick
มุมมอง 6102 หลายเดือนก่อน
UK 2024 #8 Alternative method / Trick
Interesting sum evaluated with Digamma function
มุมมอง 7812 หลายเดือนก่อน
Interesting sum evaluated with Digamma function
MIT Integration Bee 2022 Regular Season #14
มุมมอง 8552 หลายเดือนก่อน
MIT Integration Bee 2022 Regular Season #14
I fixed a mistake before it caused SERIOUS HARM
มุมมอง 1.3K2 หลายเดือนก่อน
I fixed a mistake before it caused SERIOUS HARM
This ALMOST yields a beautiful result :)
มุมมอง 1.4K2 หลายเดือนก่อน
This ALMOST yields a beautiful result :)



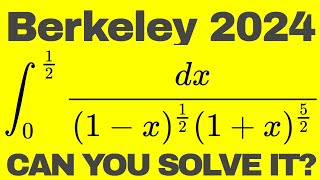




What if a was negative?
Wow, i like how it simplifies though the du initially looked daunting 👍
S=Li_-2(1/2)+2Li_-1(1/2)+3Li_0(1/2)+4Li_1(1/2) Li_1(x)=-ln(1-x) Li_s-1(x)=x•d/dx(Li_s(x)) Li_0(x)=x/(1-x) Li_-1(x)=x/(1-x)^2 Li_-2(x)=x(1+x)/(1-x)^3 S=(3/4)/(1/8)+1/(1/4)+(3/2)/(1/2)-4ln(1/2) S=6+4+3+4ln(2) S=13+4ln(2)
thanks max!
it might not be the fastest method, but it's still my favourite because it lets us solve the general case for sin(x)^n / x^n
That’s interesting. But what’s Laplace(sin(x)^n)?
@@owl3math you can find it using a power reduction formula for sin(x)^n. i found it via fourier series and residue theorem, but i believe you can do it easier with complex exponentials and binomial expansion
Excellent! Yes, it's hard to find examples where it works... But when it does it's very refreshing🍻
Yep love this formula! And as you probably know you got me interested in it again so thanks! 🍻
sin(ln(x))=(x^i-x^-i)/2i I=-i/2•int(x^(2+i)-x^(2-i))dx I=x^(3+i)/2i(3+i)-x^(3-i)/2i(3-i)+C 1/(3+i)=(3-i)/10 I=x^3/20i•((3-i)x^i-(3+i)x^-i)+C =x^3/10•(3sin(ln(x))-cos(ln(x)))+C
Nice! You can use this approach to easily get the general formula Int[ x^n sin(ln x) = x^(n+1)/( (n+1)^2 + 1) { (n+1) sin(ln x) - cos(ln x)}.
excellent!! thanks :)
Awesome solution from Waarschijn and excellent presentation!
thanks Slavino! :)
Nice, thanks!
Thank you! 😃
Very elegant👏👏👏
Love it! Great suggestion from Waarschijn
x=sinθ,I=int(1/(1+sinθ)^2)..θ=0,π/6...
Fantastic
Thanks! :)
I=int[0,1/2](1/sqrt(1-x^2)(1+x)^2)dx x=sin(t) dt=dx/sqrt(1-x^2) I=int[0,pi/6](1/(1+sin(t))^2)dt t->pi/2-t I=int[pi/3,pi/2](1/(1+cos(t))^2)dt u=t/2 dt=2du I=1/4•int[pi/6,pi/4](sec^4(u))du b=tan(u) db=sec^2(u)du I=1/4•int[1/sqrt(3),1](1+b^2)db I=(b/4+b^3/12)|[1/sqrt(3),1] I=(18-5sqrt(3))/54
thanks Max
Is the other method x = (1-u)/(1+u) ? This is another overpowered secret weapon like the Weierstrass substitution and it works very nicely on this one
Hi Samir. No but it is a substitution and video is coming soon. How would that work with (1-u)(1+u)?
@@owl3mathIf you substitute x = (1-u)/(1+u), after finding dx and everything the integral simplifies to (1/4) * (1/3 to 1)∫(u^(1/2) + u^(-1/2))du
Way to stick with it! If instead of updating the bounds with each substitution, you back substituted instead, the integral is a little nicer. It's just -(x+2) sqrt(1-x^2) / 3(x+1)^2, that's the antiderivative of the original without thinking about bounds. Plugging 0 and 1/2 into there is not so bad (at least given how the final answer is, you still have to play with some sqrt(3) in places)
Thanks David. Good idea! Just evaluating the bounds is probably the longest part so cleaning that up would save some trouble.
You did a great job! That was pretty unwieldy. It will be interesting to see how the other method compares.
thanks Mike! I just finished recording method 2 this morning and I can tell you its less work for me for sure :)
Excellent and clear video👏👏👏A lot of work... You should get some rest... 😀
Ha! Thanks Doron! You’re right I was exhausted after this one. I recorded method 2 and it’s definitely quicker but plenty of interesting things in this one. 👍👍👍
Now, This is fantastic.❤👏🏽 I did it haphazardly by assuming that n is an integer then the xfloor(x) will now be x² integrating and working out the limit gives 1/3 I think I should invest time and learn how to integrate floor and ceiling functions. Thanks for the video.
Nice thank you! Yes floor and ceiling is fun. I've done many videos on it across all the channels. :)
Very bad handwriting. Can't read '+' sign properly
The max function in the integrand was very confusing because one may not realize if it's intended to maximize the argument on the variable x or the positive integer k. Based on your clear explanation, x should be considered fixed and k variable in the computation of the max. Essentially what value k should take in order for the max argument to be maximized for a given x.
Hi Slavino. I must admit I didn't think about this at all when I was recording it and kind of did the natural thing but afterward I started thinking about it and it really is a confusing expression. Also I had some concern about my thumbnail which shows the k as separate from the max function where is MIT has it as part of the function. I'm not 100% sure if that matters but yeah confusing!
@owl3math The confusion stems from the separation of k and the max function, and your comment is correct at pointing it out. Perhaps k should have appeared right under max, making it cristal clear that the argument of the function is to be maximized based on k.
@@slavinojunepri7648 yep exactly. But I didn't know how to do that in LaTex :) I'll look into it
I don't know if you can actually do this but I got the same answer by seeing it as a limit of the the integral divided by n^3. Then noting that when n->inf both the denominator and numerator tends towards infinity so I can use L'hopitals rule and the numerator simply becomes n*floor(n) by fundemental theorem of calculus. Therefore I=lim n->inf (1/3*floor(n)/n) which I evaluated to be just 1/3👍
Nice I think that works. I believe I did it that way in another video similar to this one.
Maybe it's because I don't know enough about infinite limits, but shouldn't the summation be from 1 to floor(n), giving a residue integral from floor(n) to n? This made my answer diverge, because it goes to 0 for all non-integer values of n and goes to 1/3 for all integer values of n, which makes more sense to me, since at everywhere except the integer values, n^3 will be much larger that floor(n)^3. So I guess I just want to know why we are allowed to sum directly to n instead of summing to floor(n). Correction: Just did the limit to infinity of ((floor(n))^3)/(n^3) by splitting (floor(n)) into (n-c)^3 for some 0≤c<1, and the limit converges to 1, so my intuition was wrong, and the limitt doesn't depend on whether n is an integer. Going through the math again, this does mean that the integral converges to 1/3 for all values of n. However, it would still be nice to know why we are allowed to sum up to n directly, and not just up to floor(n).
is this for the upper bound of the sum? Like Max suggested in the comments that it should be n-1
Nice video 👍 actually you can get 1/3 just by getting the highest-degree term at 4:55 (which is (1/3)n^3) and easily get the limit =1/3. on the actual competition this can save a lot of time.
I think i see what you mean. Like don't even calculate the sum because you know you're going to be taking a limit later? Thats very nice! I can't really do that in a video but like you said it would be a good time savings in competition.
I got the samd answer even tho i made a mistake while taking the integral i forgot about the x so i just put the bounds as theyre😭
This is analogous to Abel's summation formula. Consider the sum 1/2 * \sum_{k\leq n} k^2 = n^3/2 - \int_1^n x[x] dx by the summation formula. Rearrange for the integral, divide by n^2 and apply the formula for a sum of squares. The lower bound of the integral can be 0 or 1 because of the floor function. Taking the limit as n tends to infinity we get 1/2-1/6 = 1/3.
shouldn't the upper bound of the sum be n-1?
No, since the original integral bound of n is incorporated into the sum as an assumed infinity due to the whole n-->inf limit
either that and the lower bound be 0, or the bounds of integration be k-1 and k
@ the k=0 case evaluated to 0 so he removed it
@@maxvangulik1988 I missed that mb
Nice job ; )
thanks!
I am actually quite novice when non-elementary integrals come into place. So could you please explain these integral separately? How did they come into existence and how and why do we write in a way we do? For example, dilogarithm, Si(x) etc. Thanks for such a wonderful video. Happy New Year (I know I'm late 😅) That's all for now. Stay safe and healthy. Have a good day 😊😊
Thanks! And happy new year to you! For Dilogarithm I think the notation gives us a compact way to represent it when there isn't necessarily a nice numerical value. It's nice having some labels and names for things. :)
Our beautiful dilogarithm 👏👏👏
yep love it! :) 👍👍👍
I actually was just thinking about this kind of problem with same bounds substitution but for a different integral. Though I am unqualified to give a statement, my opinion is that when we can make a "complete" substitution than there should be no problem, and by "complete" I mean a substitution that doesn't result in +-f(x) in the integrand For example integrating from -3 to 3 over x^2 and setting u = x^2 -> du =2xdx = +-2sqrt(u)dx. Thus the integral stops making sense as we are already integrating over a curve and not a function which is plainly not permitted (at least in single variable calculus)
I was thinking about the following points: Cos(x) =-cos(pi+x) so splitting the integral from o to pi of cos(x) and from pi to 2pi of -cos(pi+x) we can do a substitution for second integral to have y=pi+x so the boundaries become 2 pi to 3 pi but because of periodicity it is just the negative of the first integral so it is a fancy way of writing 0 because of the odd power exponent
Great parametrization. I thought the Feynman's trick carried out twice in the earlier evaluation was awesome, but then this turned out to be even better. Thanks to Samir and you for this excellent evaluation. Have a great 2025 ahead and continue to regale us with these lovely integrals. My question is did the UK Bee guys imagine that Frullani's integrals could pop up here? They are so enamored with the Feynman's trick.
hey Mohan! thanks! And Happy new year to you. Wish you well in 2025! :) I can't really answer your question but I will say I didn't expect the Frullani integral so its possible it would surprise UK as well.
Great insights from Samir, and excellent presentation. This channel brings some of my very distant math memories back alive, excitng and rejuvenating the brain cells in the process. Please keep up the good work.
Thanks a lot Slavino. Appreciate your nice comments and support. :)
Awesome 👍
Your parametrization suggestion is excellent, and the use of the Fullani's integral is the icing on the cake. Well done Samir!
Thanks Samir! Great idea for this one. Love the technique :)
I really enjoyed seeing the alternate use of Feynman's trick. Nice job.
Thanks Mike! There was a few nice ways to do this one. Happy New Year!
Excellent video👏👏👏 I've never encountered Frullani's Formula... Thanks for the education and Happy 2025🍻
Thanks Doron. Yes that’s a powerful formula! Happy 2025 sir! 🍻
Nice, I liked the slightly more general method shown in the book where we look at a general function d(x) instead of e^(1/x). The property d(x)d(-x)=1 makes the result obvious for any such kind of function d(x)
Thanks. Makes sense. The general formula is very nice for this one 👍
do you know if the integral of 0 to infinity of e^-(x+1/x)^2 is possible, or have you done a vid on it ?
Good one! Not too hard. It's a nice variation on the Gaussian integral. I think i want to do a video.
Worst video. Very bad handwriting. Goes very fast
Ok thanks for the input
Fantastic
thanks Slavino!
Where do you find these integrals? I sat the UK int bee 2024 this wasn't on it or is it a different competition?
Hi. I got these for UK 2024 here: www.scribd.com/document/703490462/UK-Integration-Bee-Practice-DUTIS-and-Double-Integrals
Or, you can just use the Gamma Function. I=lim(s to 0) int_(0 to inf) e^(-x)*x^(s-2)*(3x-1+e^(-3x))dx, int_(0 to inf) e^(-x)*x^(s-2)*3x dx= 3*Gamma(s), int_(0 to inf) e^(-x)*x^(s-2)dx=Gamma(s)/(s-1), int_(0 to inf) e^(-x)*x^(s-2)*e^(-3x) dx= 4^(1-s)*Gamma(s)/(s-1), so I=lim(s to 0) (3+1/(1-s)-4^(1-s)/(1-s))*Gamma(s), when s approachs to 0, Gamma(s)=1/s, 1/(1-s)=1+s, 4^(1-s)/(1-s)=4+4*(1-ln4)s, so I=4ln4-3. You can also use the same method to solve the other integral you mentioned.
Hi. I follow you up to this point: I=lim(s to 0) (3+1/(1-s)-4^(1-s)/(1-s))*Gamma(s) but then this is an indeterminate form. How did you evaluate this to 4ln4-3?
@owl3math That indeterminate form is easy to evaluate using series expansion. First, you need to know the series expansion of Gamma(s) at s=0, which is Gamma(s)=1/s-r+O(s), where r is Euler-Mascheroni constant, but here we only need the first term, i.e Gamma(s)=1/s, because all the other terms will result to 0 anyway. And Gamma(s)=1/s when s approachs to 0 is easy to prove using s*Gamma(s)=Gamma(s+1), since s approachs to 0, s+1 approachs to 1, Gamma(s+1) also approachs to 1, so Gamma(s)=1/s. Now all you need is the Taylor expansion of 3+1/(1-s)-4^(1-s)/(1-s), and since all the s^2 terms and higher terms will result to 0, you'll only need the constant term and s term, which is very easy to calculate. which means 1/(1-s)=1+s, 4^(-s)=1-s*ln4, 4^(1-s)/(1-s)=4*(1-s*ln4)*(1+s)=4+4*(1-ln4)s, 3+1/(1-s)-4^(1-s)/(1-s)=(4ln4-3)*s, so the indeterminate form is equal to 4ln4-3.
@@toadjiang7626 nice! Thanks for the explanation :) 👍
Perfecto👏👏👏 Loved the didactic step-by-step approach🍻
and thank you for the idea! :) 👍👍👍
Interestingly enough...you could also use feynman's technique by letting I (t) = the integral given but with e^(-xt) replacing e^(-x)( where t>0).....then by differentiating twice and integrating twice and letting t =1 we get the answer 4ln4 -3 = ln 256 -3
Nice! That was the way I did the other one I mentioned at the beginning of the video. I think it was this one: th-cam.com/video/49R9ukx0B00/w-d-xo.htmlsi=7mrWFybP69ScedNn
@owl3math yup exactly....
@@owl3math for your next integral video can you solve the integral of (ln (cos x))^2 from zero to pi/2...try it using complex analysis the contour and the final result is really nice...
@@DihinAmarasigha-up5hf - sorry I generally can't take requests but I will keep it in mind for some day in the future.
Cool. You could also namnyeF it by noting that the target integral is - d/da Int[ e^(-a x)].
Yep good point! You could namnify it
u=e^x du/u=dx I=int[1,2](ln(u)/u^3)du U=ln(u) dv=du/u^3 dU=du/u v=-1/2u^2 I=-ln(2)/8+1/2•int[1,2](u^-3)du I=-ln(2)/8+3/16
Excellent👏👏👏 Almost what I meant... Will send a slightly modified version by email 🍻
Nice thanks! Had a feeling your way could be a little different :)
Excellent
thanks Slavino!
Unit step function feels like Dirac delta function?
closely related. Unit step function is the integral of dirac delta or dirac delta can be considered like a derivative of the step function.
i still can't even begin to comprehend how some people think of these solitons
Hi Lemon. Yes it’s a nice method!