A Nice Algebra Problem | Math Olympiad | Can you solve it for x?
ฝัง
- เผยแพร่เมื่อ 10 ก.พ. 2025
- math olympiad
olympiad math
math olympiad question
math olympiad questions
math olympiad problem
math olympiad problems
olympiad math problems
algebra olympiad problems
math
maths
algebra
algebra problem
nice algebra problem
a nice algebra problem
equation
exponential equation
a nice exponential equation
radical equation
a nice radical equation
math tricks
imo
Find the value of x?
How to solve 8^x - 2^x = 120
In this video, we'll show you How to Solve Math Olympiad Question A Nice Algebra Problem 8^x - 2^x = 120 in a clear , fast and easy way. Whether you are a student learning basics or a professtional looking to improve your skills, this video is for you. By the end of this video, you'll have a solid understanding of how to solve math olympiad exponential equations and be able to apply these skills to a variety of problems.
#matholympiad #maths #math #algebra
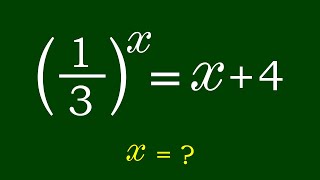








With complex roots (I hope this is correct):
8^x - 2^x = 120
(2^3)^x - 2^x = 120
2^(3x) - 2^x = 120
2^(x*3) - 2^x = 120
(2^x)^3 - 2^x = 120
Let u = 2^x
u^3 - u = 120
u^3 - u - 120 = 120 - 120
u^3 - u - 120 = 0
u^3 + (-25u + 24u) - (24 * 5) = 0
(u^3 - 25u) + (24u - [24 * 5]) = 0
u(u^2 - 25) + 24(u - 5) = 0
u(u^2 - 5^2) + 24(u - 5) = 0
u(u + 5)(u - 5) + 24(u - 5) = 0
(u - 5)(u * [u + 5] + 24) = 0
(u - 5)(u^2 + 5u + 24) = 0
Suppose u - 5 = 0
u - 5 = 0
u - 5 + 5 = 0 + 5
u = 5
Remember, u = 2^x
2^x = 5
log(2^x) = log(5)
x * log(2) = log(5)
x * log(2) / log(2) = log(5) / log(2)
x = log_2(5)
x1 = log_2(5)
Suppose u^2 + 5u + 24 = 0
1 * u^2 + 5 * u + 24 = 0
Let a = 1, b = 5, c = 24
u = (-b +/- sqrt[b^2 - 4 * a * c]) / (2 * a)
u = (-5 +/- sqrt[5^2 - 4 * 1 * 24]) / (2 * 1)
u = (-5 +/- sqrt[25 - 96]) / (2)
u = (-5 +/- sqrt[-71]) / 2
u = (-5 +/- sqrt[71 * (-1)]) / 2
u = (-5 +/- sqrt[71] * sqrt[-1]) / 2
u = (-5 +/- sqrt[71] * i) / 2
Remember, u = 2^x
2^x = (-5 +/- sqrt[71] * i) / 2
ln(2^x) = ln([-5 +/- sqrt(71) * i] / 2)
x * ln(2) = ln([-5 +/- sqrt(71) * i] / 2)
x * ln(2) / ln(2) = ln([-5 +/- sqrt(71) * i] / 2) / ln(2)
x * 1 = ln([-5 +/- sqrt(71) * i] / 2) / ln(2)
x = ln([-5 + sqrt(71) * i] / 2) / ln(2), or x = ln([-5 - sqrt(71) * i] / 2) / ln(2)
x2 = ln([-5 + sqrt(71) * i] / 2) / ln(2)
x2 = log_2([-5 + sqrt(71) * i] / 2)
x2 = log_2(-5 + sqrt[71] * i) - log_2(2)
x2 = log_2(-5 + sqrt[71] * e^[i * tau / 4]) - 1
x3 = ln([-5 - sqrt(71) * i] / 2) / ln(2)
x3 = ln(-1 * [5 + sqrt(71) * i] / 2) / ln(2)
x3 = ln(-1) / ln(2) + ln([5 + sqrt(71) * i] / 2) / ln(2)
x3 = ln(e^[i * tau / 2]) / ln(2) + log_2([5 + sqrt(71) * i] / 2)
x3 = (i * tau / 2) * ln(e) / ln(2) + log_2(5 + sqrt[71] * i) - log_2(2)
x3 = (i * tau / 2) * 1 / ln(2) + log_2(5 + sqrt[71] * e^[i * tau / 4]) - 1
x3 = i * tau / (2 * ln[2]) + log_2(5 + sqrt[71] * e^[i * tau / 4]) - 1
{x1, x2, x3}
=
{
log_2(5),
log_2(-5 + sqrt[71] * e^[i * tau / 4]) - 1,
i * tau / (2 * ln[2]) + log_2(5 + sqrt[71] * e^[i * tau / 4]) - 1
}
Outstanding Solution 👌👌👌
Very nice! ❤
Thank you so much for more uploads youtube videos like mathematics
More to come! ❤
Let 2^x=y
Y³-y=120
Y³-y-120=0
Y³-y-125+5=0
(Y³-5³)-(y-5)=0
(Y-5)(y²+5y+25)-(y-5)=0
(Y-5)(y²+5y+24)=0
Case 1
Y-5=0
Y=5
2^x=5
Log2^x=log5
Xlog2=log5
X=log5
²
Case 2
Y²+5y+24=0
Delta=5²-4.1.24=25-96
=-71
Very nice! ❤
Godbless You sir watching from Philippines 🙏🙏🙏
Thank you so much! ❤
I like this problem.
Thanks for liking! ❤
y^3-y=125-5
y^3-125 - (y-5)=0
(y-5)(y^2+5y+25)=0
y has 1 real root (y=5, then x=Ln5) and 2 complex roots (which can easily be found using the quadratic formula, then 2 complex logarithmic roots from here).
Very nice! ❤
Math Olympiad: 8ˣ - 2ˣ = 120; x =?
8ˣ - 2ˣ - 120 = 0, [(2ˣ)³ - 5³] - (2ˣ - 5) = (2ˣ - 5)[(2ˣ)² + 5(2ˣ) + 25 - 1] = 0
(2ˣ - 5)[(2ˣ)² + 5(2ˣ) + 24] = 0, 2ˣ > 0; (2ˣ)² + 5(2ˣ) + 24 > 0
2ˣ - 5 = 0, 2ˣ = 5, x = log₂5 = 2.322
The calculation was achieved on a smartphone with a standard calculator app
Answer check:
x = log₂5 = 2.322, 2ˣ = 5: 8ˣ - 2ˣ = (2ˣ)[(2ˣ)² - 1] = 5(25 - 1) = 120; Confirmed
Final answer:
x = log₂5 = 2.322
Very nice! ❤