A Special Differential Equation | Inspired by
ฝัง
- เผยแพร่เมื่อ 17 ม.ค. 2025
- 🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @sybermathshorts
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
Subscribe → www.youtube.co... (shorts)
⭐ Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
🤩 The inspiration for this video comes from a video made by @blackpenredpen:
• Derivative of the Lamb...
😉 Check out this graph from desmos:
www.desmos.com...
#CalculusProblems #DifferentialEquations #Calculus
via @TH-cam @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus
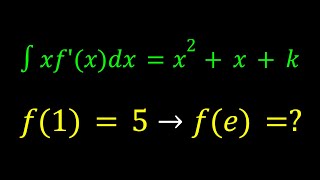








I have never studied maths all the knowledge I have is from high school! I am able to solve most of your problems. This one was not in my sphere of knowledge but you make everything so easy! I wish you were my teacher!! Thank you❤❤❤❤❤
Excellent! Thank you for the kind words!!! 💖🥰
If you take absolute value into consideration i.e. integration of (1/y) dy is ln(abs(y)) + C, then my answer is coming as:
y = W_n (k * abs(x) * sign(y))
Where sign(y) is 1 if y > 0, else -1 if y < 0.
W_n is n-th branch of Lambert-W function.
BPRP loves that function. He uses fish as variables!
I know! His video gave me the inspiration
Still had to use Lambert W-function!)) It seamed that you didn't like it))
It's inevitable! 😲😜😄
Very nice ODE with the Lambert W function. I have never seen this before. Thanks a lot👌
Glad you liked it!
You can write the solution in a more simple way with x = x(y) = C y exp(y).
Thanks sir , though I am a physics student but i love your videos , i really appreciate about your knowledge ,...
All the best
Lambert W function worked in this equation. 👍🏻👍🏻👍🏻👍🏻
A wild Lambert W appears! Yay!
😍
Differential equation question - separable type - necessary information, knowledge of integration methods - solve x and y alone ;
dy/dx=(1/x)(y/(1+y))
integral(((y+1)/y)=integral(1/x)
ln(ye^y)=ln(Cx)
Ye^y=Cx
Very good
Cool, but how were you able to turn the c into an ln(k)?
A constant can be written as ln of another constant. For example, 2 can be written as ln(e^2) and -1 can be written as ln(1/e). Soo if k=e^c then c=ln k
@@chaithanyab8007 OK thanks - I just arrived at that same constant differently.
It’s an arbitrary constant. It can be whatever makes your derivation nicer
It is a separable de, no problem
Haaaaaa finally Lambert's function apears
It's inevitable! 😲😜😄
I don't know what even is Lambert function I'm too small to understand XD.
I didn't know it, either, before I started this channel but my viewers kept talking about it and then I saw the video from @blackpenredpen and I decided to make this video.
Separable ode
Only difficulty is calculating integrals
W nedir? Onu bilmediğim için soruyu çözemedim. W'ya kadar geldim, sonrasını anlamadım.
W is the Lambert function
W(xeˣ)=x denklemini saglayan fonksiyon yani f(x)=xeˣ in tersi
x=kWy)....ho invertito le variabili...y=kW(x),dovrebbe essere corretta
y+lny=lncx
Yes,which is ln(e^y y)=ln(cx) so y e^y=cx and you apply lambert function on both sides and get y=W(cx). Lambert function is bprp favorite function😀
@@yoav613 i got these pieces by the chatting space
y=W(kx)
Yess! That's how this video was inspired
y= 0,5y^2/x(y+1); y''=1