CD and GH are respectively the bisectors of angle ACB and angle EGF | Exercise 6.3 class 10 Q10

Exercise 6.3 Question 10 Class 10
or
Exercise 6.3 class 10 Question 10
CD and GH are respectively the bisectors of ∠ ACB and ∠ EGF such that D and H lie on sides AB and FE of Δ ABC and Δ EFG respectively. If Δ ABC ~ Δ FEG, show that:
(i) CD/GH =AC/ FG
(ii) Δ DCB ~ Δ HGE
(iii) Δ DCA ~ Δ HGF
HINT of Q10 of exercise 6.3 class 10
If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar
Read it :
INTRODUCTION
1.Two figures are said to be congruent, if they have the same shape and the same size.
2.Two figures having the same shape (and not necessarily the same size) are called similar figures.
3.All congruent figures are similar but the similar figures need not be congruent.
4.Two polygons of the same number of sides are similar, if (i) their corresponding angles are equal and (ii) their corresponding sides are in the same ratio (or proportion)
Note📝
The above question is taken from NCERT class 10 chapter Triangles Exercise 6.3 Question 10.
Practice :
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that Δ ABE ~ Δ CFB.
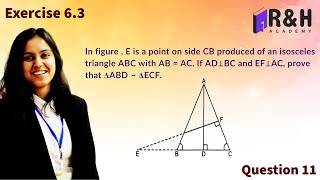
• E is a point on the si...
Revise✍🏻:
Circles:
• Circles- class 10th NCERT
Real Numbers:
• Real Numbers - Class 10th
Polynomials
• Polynomials- Class 10th
Quadratic Equation
• Quadratic Equations- C...
Trigonometry
• Trigonometry - Class 10th
Connect with us 🤩:
INSTAGRAM: ...
FACEBOOK: / rndh.academy
#Triangles #class10maths #ncert