
- 235
- 375 551
Silver
United States
เข้าร่วมเมื่อ 2 ก.พ. 2020
My academic immature-ness side of me.
Integration Bee Tricks
Math Equations
Other-ish...yep
Integration Bee Tricks
Math Equations
Other-ish...yep
วีดีโอ
The integral that nearly killed me...
มุมมอง 3042 ชั่วโมงที่ผ่านมา
During an integration bee, I had two minutes to solve this integral. While I was speed integrating this integral, something went wrong and my integral felt a little too easy or I noticed my answer was very unfamiliar and didn't seem quite right. I didn't wanna risk it, so I decided to erase everything and speedsolve my integral again with 45 seconds remaining. Down to the last second, I managed...
The Integral that Got Me Eliminated...
มุมมอง 4172 ชั่วโมงที่ผ่านมา
Background: I was competing at my first integration bee at a university when I was in 12th grade. I got eliminated at semi-finals and this was the integral that screwed me over.
I Tried Caltech Math Meet 2024 Integration Bee Finals
มุมมอง 1.1K2 ชั่วโมงที่ผ่านมา
Problems: www.caltechmathmeet.org/problems/2024 I think this year is a bit easier. Last year problems was chaos lol.
I Attempted Caltech Math Meet 2024 Integration Bee Qualifying Exam
มุมมอง 1.1K7 ชั่วโมงที่ผ่านมา
Actual Score: 9/14 ;_; If I was more accurate and serious: 12/14 CMM 2024 QUAL Exam: www.caltechmathmeet.org/_files/ugd/006423_c9d6264aa8d348c5a7b2536704204f91.pdf
3 Potential Methods to Survive this Evil Integral!!
มุมมอง 7019 ชั่วโมงที่ผ่านมา
Thank you CBMiami and Ninad for these potential suggestions! Steps to practice a certain method: Step 1: First solve the integral with that method untimed to understand the process. Step 2: Speedsolve the integral with that method. Do it 3 times trying to get faster. Step 3: Once your brain feels annoyed, it will skip steps and memorize the next step integral expression. Speed integrate by skip...
I Attempted Harvard MIT Math Tournament Integration Bee Feb 2024 Qualifying Exam!!!
มุมมอง 2.4K14 ชั่วโมงที่ผ่านมา
I am very very sad. Not only because I scored 12/20 but because all archives of HMMT Integration Bee are outdated and no longer exists. ;_; Thank you still to Edward Jin for the archives. Integrals I got correct: 1,4,5,6,7,8,9,10,11,13,16,18. I'm never using a timer on Google again...
You MUST Memorize the Process of this Evil Integral!!!
มุมมอง 90116 ชั่วโมงที่ผ่านมา
This integral looks small and simple to do, but it is horrific to speedsolve, especially under 2 minutes!! Can you solve this faster??? Send me a vid or send me your solution in the comments! Thanks for watching! Latex: \int_{0}^{1} \sqrt{x \sqrt{x}}\;dx
Harvard MIT Math Tournament Integration Bee SPRINT Training Practice
มุมมอง 1.1K21 ชั่วโมงที่ผ่านมา
Were you able to catch up with me? If you're able to speed integrate faster than me throughout the whole video, you're certainly more than ready for HMMT Integration Bee!! I wish you HMMT contestants the best of luck!! HMMT Integration Bee Topics List here: artofproblemsolving.com/community/c5h3108982_hmmt_integration_bee_topics
Huge Important Tips for Practicing Mock Integration Bees!!
มุมมอง 289วันที่ผ่านมา
Scranton University Integration Bee to Practice Accuracy: www.scranton.edu/academics/cas/math/bee.shtml Mock Integration Bees (Quite Outdated ;_; so some you might just need to search): sites.google.com/view/silveralchemist/integration-bee-stuff/integration-bee-mock-problems
Integration Bee Tricks Tier List 2 (Most Helpful)
มุมมอง 2.7Kวันที่ผ่านมา
Timestamp: 0:54 - Symmetry 2:22 - Area of a Partial Circle 5:54 - Gamma Factorial 8:17 - Wallis' Trick 10:05 - Hyperbolic Manipulation 11:32 - Hyperbolic Substitution 12:13 - Forced U-substitution 13:58 - Reverse Product Rule/Reverse Quotient Rule 15:53 - Loophole Log 17:33 - Heaviside Method 19:16 - Zero Substitution 21:56 - Trigonometric Manipulation 24:24 - Advanced Trig-Substitution 26:40 -...
Integration Bee Tricks Tier List (Most Satisfying/Coolest Techniques)
มุมมอง 6Kวันที่ผ่านมา
Tier List: tiermaker.com/create/speed-integration-tricks-17551488 Weaponizing the Integral Trick: arxiv.org/pdf/2201.12717 Timestamp: 0:51 - Caveman Trick 2:28 - Advanced Trig-Substitution 4:25 - Ahmed's Substitution 7:17 - Area of a Partial Circle 7:34 - Symmetry 8:03 - Beta Function 8:38 - British Substitution 10:04 - Chebyshev Caveman (Chebyshev Binomial Integral Formula) 13:12 - Complexifyi...
10 Most Unfair Integrals from MIT Integration Bee
มุมมอง 4.7K14 วันที่ผ่านมา
0:47 - A Disgusting Semi-IBP/Reverse Quotient Rule (MIT 2006) 2:36 - A Nasty Trig Integral (MIT 2022) 3:33 - A Nasty Cube Root Reverse Product Rule (MIT 2022) 4:28 - Orthogonality Troll??? (MIT 2022) 6:43 - A Confusing Piecewise Integral (MIT 2022) 7:38 - A Double Summation Integral (MIT 2022) 9:30 - Glasser's Master's Corollary??? (MIT 2022) 11:35 - MODULUS!!? (MIT 2022) 15:07 - Representation...
Integration Bee Training for Advanced #20.3 - A Tangent Corollary to Glasser's Master Theorem
มุมมอง 313หลายเดือนก่อน
Memorable Timestamp: 1:00 - The Corollary Explanation 6:59 - An MIT Integral Example 8:00 - Practicing Recognition/Manipulation 9:04 - A Familiar Integral 10:32 - A Sneaky Hybrid Example
Integration Bee Training for Advanced #20.2 - Full Power of Glasser's Master Theorem
มุมมอง 246หลายเดือนก่อน
Memorable Timestamp: 0:14 - The FULL Concept 3:57 - A Straightforward Example 5:18 - MAKE SURE ITS MONIC!! 7:27 - Partial Fraction Check 8:50 - A Horrifying Integral Example 12:31 - Another Intimidating Example
Integration Bee Training for Advanced #20.1 - Intro to Glasser's Master Theorem
มุมมอง 230หลายเดือนก่อน
Integration Bee Training for Advanced #20.1 - Intro to Glasser's Master Theorem
Integration Bee Training for Advanced #19.2 - Constructive Feynman Technique
มุมมอง 241หลายเดือนก่อน
Integration Bee Training for Advanced #19.2 - Constructive Feynman Technique
Integration Bee Training for Advanced #19.1 - Introduction to Feynman Technique!!
มุมมอง 520หลายเดือนก่อน
Integration Bee Training for Advanced #19.1 - Introduction to Feynman Technique!!
Integration Bee Training for Advanced #18.2 - Trickier Integrals with Euler's Reflection
มุมมอง 289หลายเดือนก่อน
Integration Bee Training for Advanced #18.2 - Trickier Integrals with Euler's Reflection
Integration Bee Training for Advanced #18.1 - Introduction to Euler's Reflection
มุมมอง 233หลายเดือนก่อน
Integration Bee Training for Advanced #18.1 - Introduction to Euler's Reflection
Integration Bee Training for Advanced #17.6 - MAZ Identity
มุมมอง 170หลายเดือนก่อน
Integration Bee Training for Advanced #17.6 - MAZ Identity
Integration Bee Training for Advanced #17.5 - Laplace of Integral Functions
มุมมอง 151หลายเดือนก่อน
Integration Bee Training for Advanced #17.5 - Laplace of Integral Functions
Integration Bee Training for Advanced #17.4 - Special Laplace Formulas!!
มุมมอง 163หลายเดือนก่อน
Integration Bee Training for Advanced #17.4 - Special Laplace Formulas!!
Integration Bee Training for Advanced #17.3 - Laplace Integration Property
มุมมอง 198หลายเดือนก่อน
Integration Bee Training for Advanced #17.3 - Laplace Integration Property
Integration Bee Training for Advanced #17.2 - Laplace Differentiation Property
มุมมอง 191หลายเดือนก่อน
Integration Bee Training for Advanced #17.2 - Laplace Differentiation Property
Integration Bee Training for Advanced #17.1 - Introduction to Laplace Transforms!!
มุมมอง 287หลายเดือนก่อน
Integration Bee Training for Advanced #17.1 - Introduction to Laplace Transforms!!
Integration Bee Training for Advanced #16.6 - Tricky Limit Substitutions
มุมมอง 158หลายเดือนก่อน
Integration Bee Training for Advanced #16.6 - Tricky Limit Substitutions
Integration Bee Training for Advanced #16.5 - Limit Substitution!!
มุมมอง 192หลายเดือนก่อน
Integration Bee Training for Advanced #16.5 - Limit Substitution!!
Integration Bee Training for Advanced #16.4 - Trickier Domain Splitting
มุมมอง 331หลายเดือนก่อน
Integration Bee Training for Advanced #16.4 - Trickier Domain Splitting
Integration Bee Training for Advanced #16.3 - Introduction to Domain Splitting
มุมมอง 287หลายเดือนก่อน
Integration Bee Training for Advanced #16.3 - Introduction to Domain Splitting






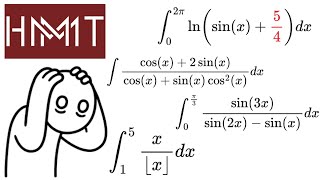


One of my favourite examples of what you've called the reverse doppelganger trick is the integral of sin(x)/(sin(x) + cos(x)). I saw it in an old STEP paper once, definitely had nice combinations of I+J and I-J!
7:49 Fellow DI method user
W
I3=-i/2•int(e^(5+3i)x-e^(5-3i)x)dx =-i/2•(e^(5+3i)x/(5+3i)-e^(5-3i)x/(5-3i))+C =-i/68•((5-3i)e^(5+3i)x-(5+3i)e^(5-3i)x)+C =e^5x/68i•(5(2isin(3x))-3i(2cos(3x)))+C =e^5x•(5sin(3x)-3cos(3x))/34+C
for the second problem, can you instead multiply top and bottom by 1/x, then you get 1/(x^(1/2))((1/x)+1), then make a u sub for (1/x+1) which reduces the integral to 1/u, therefore you get -ln((1/x)+1)?
That will not work no
@@Silver-cu5up ohhhh my bad, I forgot it's -1/x^2 and not -1/x^(1/2), thanks
In which integral competitions did you competed? And what are your results?
@@Ex-ws2vb I competed in Fresno State Central Valley Integration Bee and Reedley College. First time at Fresno State I got eliminated at semifinals. 2nd time at Reedley College and won 1st. (I didnt score perfectly tho ;_;) 3rd and 4th time at Fresno State and won both as 1st place with perfect scores
7:57 you forgot the second from chain rule making it a 9...
AAAAHHHHHHHHHHH!!!!! I swear my brain just feels lazier and lazier when it comes to speed bashy integrals ;_;
I caught that too
I just got the point of all the solution at 3:59 👍
I did it fast and got mostly the right answer. I don’t get the |x| part though. At the start of the integral there’s no mod so why is it there at the end. Oh… the square root…
The |x| is mainly for logs. The sqrt(x)'s can actually be left alone, but for logs we need to put |x| on it.
@@Silver-cu5up This isn't true in this case. The domain of this function is already x>0 because of the square root, you don't need the absolute value in the log
@@ninadmunshi2879 Ooohhhhhhhhh, I see what you mean now. Gotcha, yea becuz of the domain of sqrt(x) stops it from passing through negative side, so the |x| is not needed ever in this integral.
Satisfying
After i saw this i was thinking same as first up until you sub sec, sub cosh, use exponential definition. Slow but i understand it because im only at the end of first year.
I made a u=lnx sub and then integration by parts felt faster to me but it's probably cuz i don't speed solve and i could mess up without a u sub
@@ben_adel3437 just be very careful with that sqrt(e^u) when doing IBP very fast
Why am I learning about the periodic rule just now?
its just not that common lol, but for fractional parts its super useful and quick
This reminds me of that one time when I was in my physics class and I am that guy with the aura, the guy that teacher trusts will get the correct answer. One day we were studying YDSE (Young's Double Slit Experiment) and there was the formula for the fringe width which was β=Dλ/d, when we were deriving the formula for β we made the use of property that since the slits are so thin they are comparable to wavelength of light so theta is small and we can write sin theta as tan theta, pretty basic stuff. He also warned us that in 95% of the questions if wavelength is smaller than the distance between the slits then we can directly use β=Dλ/d, after that we did some questions. On one of the questions he threw a curved ball, he said that d=1mm, D=1m and λ=0.5mm, for the past 3-4 questions the λ was in angstrom so we directly used β=Dλ/d but here since the distances between slit (d) and wavelength (λ) were both comparable we couldn't use the formula directly. Sir told everyone to do the question and for some reason he especially called me on the board and I dont know what in the world was going on in my head at that time but I used β=Dλ/d directly and in the class of 40-50 students almost 3/4th class visibly changed their answer after seeing what I did on the board. Sir scolded me and told the entire class that dont trust anyone so easily and always trust yourself and that day was so hilarious and kinda sad because after the class was over everyone came to me one by one and said "I didn't expect this from you" "You lost your touch" and all as if I wasn't a human and I couldnt make mistakes. Moral of the story: Always check your work after you are done.
oooof but i do wish some classmates were more "human" minded rather than having this "smart boi" mask over us and have expectations. Sorry u had to go through that, but absolutely check your work after finishing
t=sqrt(x) dt=dx/2sqrt(x) I=2•int(ln(t^2))dt I=4t(ln(t)-1)+C I=2sqrt(x)ln(x)-4sqrt(x)+C
very slick way for those who are more comfortable with forced u-sub and memorized the integral of ln(x)
You have taught me a great deal about Integration sir. Please keep these videos up, they are truthfully fantastic!
I don’t think that Integral 7 even converges lol. The integrand should have a vertical asymptote at x=0.
OMG UR RIGHT LMAO
Hi Silver, thank you so much for making this series! I've had so much fun and I've learnt so much. Do you have any recommendations for resources on learning Feynman's trick or infinite series?
UK University Integration Bee has a lot of integrals that utilizes Feynman Technique and Taylor Series. You could also search on MathStackExchange, but other than that there's not much resources that has a convienent list.
Ive seen in you videos you talk about ninja sub and caveman sub and all these techniques. Where did you learn them all, and do you can where someone could go to learn them all fast? Also where did you learn to integrate these discrete functions? Like that floor and {x} function, and the max and min.
I second this question!
Majority was self-taught and archiving integration techniques from other people's solutions. Unfortunately there was no resources for any of these tricks, so I decided to kind of popularize them and give it a name to help memorize and practice such techniques. The piecewise functions like floor(x), {x}, and max(x), I was kind of trained by my friends on how to solve those types of integrals, and then Brilliant.org helped me generalize a method on how to speedsolve them (unfortunately the page doesn't exist anymore ;_;)
wow
"do not be like me" 12:07 ... But what if I want to?🥲
Become a better me xD
your pen strokes got me bricked bro icl
@@SCBA-if4wl LMFAO
arctan(sqrt(2)+1) has a known value 😈
1+sqrt(2) is the height of a regular octagon with side length 1. Assuming the points are labeled A-H going counterclockwise, construct triangle ABE. The height of this triangle is sqrt(2)+1, and the width is 1. Since line AE bisects angle HAB, which measures 3pi/4 because it's a regular octagon, angle EAB measures 3pi/8, except that's exactly the angle we want. Thus, arctan(sqrt(2)+1)=3pi/8.
@@maxvangulik1988 OMFG, THATS CRAZY!!!
@@maxvangulik1988 I feel like this must be some part of their intention when creating this problem!!! So the actual answer to that original integral would've been 3pi/4!!!
got 13/14 (I fvked up the partial fractions one hard) in 10mins and 15s so yippieee
@@adityavsx sheeeeeeeeeeesh, very nice!
@ would u be knowing any resources for algebra like you know so many for calc? I’m prepping for JEE Advanced and math is kind of??my strongest point and hence I need to sharpen it more so…
@adityavsx oh dang, unfortunately idk much resources for JEE Algebra ;_; Id probably look into those JEE videos on TH-cam where coaches do oneshots or problem marathon like Vedantu or other coaches. But if those dont help, sorry idk much about it ;_; You could also look into a book called JEE Algebra Booster (i forgot the author name but its a blue book). Thats as much as i know sadly. Sry about that.
@ no problems uff, chillax 😂thnx doe
you are very good wow, how did you initially learn all of what you know about math and speed integration to begin with? Did you study it at college/university or are you self taught?
@@fxrce6929 self-taught ;_; There werent that much resources back then sadly...so i started writing a journal to collect integration bee techniques and archive common sneaky integrals during high school.
At 7:58 the answer is correct though? 1/2(tan(π/2-1)+tan(1)-π/2) is exactly equal to that integral
CMM says it should be csc(2)-pi/4 ;_;
@Silver-cu5up That's the same thing as well 🤩 Well I guess they wanted the simplest possible form...
@@eggcorpprod really? Huh, last time i put it in wolframalpha, it was a different numerical answer
Cover up method for partial fractions ftw for Q11
@@calcul8er205 shouldve speedsolved passively 😭
One way to get around the + C is to apply a definite integral (instead of an indefinite) on both sides when you have I'(n) = some nice expression; you can choose your upper bound as the value you want, and the lower bound as a known value on the function, then add it to the other side Applying indefinite integrals can get us some pretty formulas, however applying definite will help for speed. Also for some very tricky ones applying a definite integral is actually necessary
DONT PANIC DONT PANIC DONT PANIC😊
Hey man, I recently found your channel, and I'm loving the Integration Bee content! I know there are many integration techniques- Could you ever cover examples of the more advanced ones, like Infinite Series and the Gamma Function? I think it would be great content for the channel, and offer educational value for learners such as myself. Currently a young college student, hoping to improve his skills :)👌🏽
If you go to my channel playlist, I have an Integration Bee Training Series in all 3 levels!! 😃 I believe the Gamma function is in Intermediate level, but the Infinite Series is in Advanced level. Thank you, I really appreciate it!!!
@@Silver-cu5upThe pleasure is mine, man- You're doing a great job with the content quality! 100% eager to continue watching, and I hope you use your passion to bring new ideas for future videos. 💯💜
4:26 ruined my asmr bruh
lmfao
I think the panicking makes you realized how short 10 minutes are xD
Surprisingly yea xD
in 54:15 instead of adding +x-x you could have just done +1-1 the fraction would be (x^2-1+1)/(x+1)=(x^2-1)/(x+1)+1/(x+1)=x-1+1/(x+1) (difference between two squares)
I started doing that a lot more now that I'm more advanced xD you'll even see me do this more often in advanced level integrals lol
What are the applications for these integrals? I'm wondering where they came from and what they're actually used for. Anyways, thanks for the amazing uploads, I'm thinking of hosting an integration bee myself at the community college I go to!
oh shizz!! I hope it goes well!! This integral is one of the infamous integrals known for eliminating a lot of competitors. There's not exactly an application for this integral, it just reoccurs a lot in intermediate-advanced level integration bees.
@@Silver-cu5up I see, thank you!
it's used in physics a lot but I only used it in physics so far, I don't know about others besides maths
@khanggamr7454 sounds about right lol
in 41:31 there is a nice identity that tan^-1(1/x)=cot^-1(x) and the derivative of cot^-1(x) is just -1/(1+x^2) which is waaaaaay easier than what you did
that works too!!!
I just realized that your voice is pretty good for asmr, I didnt even notice I was doing my homework while listening to you.
@@cdkw2 LMAO, someone has told me that before XD
How the upper bounce 3/2 and lower bounce 1/2 ? Kindly explain. Thank you.
the upper bounds are from 1/2 to 3/2 because w = u+1/2. Our bounds were from 0 to 1 before, so with our subsitution we get our new bounds 0+1/2 = 1/2 and 1+1/2 = 3/2.
I would really appreciate if you took more time to explain the trick, why it works, and how to use it. Maybe you would have to break up the tierlists into multiple videos but your content would connect with a larger audience. Otherwise great video. 👌
@@TM-ln5mr Most are already fully explained in my integration bee training series!! 😁
been watching the mit bees for a while now and then discovered ur channel. love it. prepping atm to attend mit and compete (hopefully) equipped with ur videos lol
@@Jolobee-x7c oh dayum, good luck!!
19:11 The plot twist goes crazy 😭
This really made me so mad ;_;
can you explain the first question please?
@@jonathanalvarado8007 its just a wacky integral using a different variable. Its pretty much an integral of x^2 dx from 0 to 1. But instead they used d' to confuse the competitors.
That twist at the end, with the arccosh 5/4, is so beautiful, i can't stop smiling.
I was genuinely astonished and devastated xD
Can I mail you the problems from Bonn maybe? Because I have no idea how the aops thing works
@@esp-elagogisch-sozialepart9701 That works yea!!
oh I just realized TH-cam doesnt do the email setting on my end, but u can just go ahead and send it here: silveralchemist544@gmail.com
t=tan(x) dt=sec^2(x)dx int((1+2t)/(1+2t/(1+t^2)))dt =int((t^2+1)(2t+1)/(t+1)^2)dt =int(1+2t^3/(t+1)^2)dt u=t+1 du=dt =tan(x)+2•int((u-1)^3/u^2)du =tan(x)+2•int(u-3+3/u-u^-2)du =tan(x)+(tan(x)+1)^2-6(tan(x)+1)+6ln|tan(x)+1|+2/(tan(x)+1)+C =tan^2(x)-3tan(x)+6ln|tan(x)+1|+2/(tan(x)+1)+C
I think inverse jailbreak is the fastest and simplest method to solve this integral. You quickly get that I = ( rectangle of area sqrt(2) ) - int y^2 + 1/2 - sqrt(y^2 + 1/4) dy (yes, minus, you have to take the minus root in the quadratic equation to satisfy f(0) = 0) from 0 to sqrt(2)
@@ninadmunshi2879 idk if it would be the simplest. The quadratic is too tricky to deal with for some others (although very satisfying), and the negative can be easily missed. And then the sqrt(x^2+1/4) theyd have to deal with after.
@Silver-cu5up I can understand the hesitation behind quadratic formula, but there's no way around this integral without some kind of "trick" and quadratic formula is a one and done instead of chugging through hordes of identities. Second, the integral of sqrt(x^2+a^2) is the area under a hyperbola. Either you can quickly do the cosh^2t integral or it's just muscle memory because this integral shows up all the time. But that means computation wise there's only two things to do that require work instead of multiple things.
Did you try the BMT? It was pretty good bprp was a gust and co host!
@@cdkw2 Im a problem writer for the BMT Integration Bee xD. This year 2024 tho i was assisted by a lot more people, so idk the official integrals and I dont have the problem sheet yet ;_;
@@Silver-cu5up ooooh npnp
can u not find exact value for integral 20?
@@fxrce6929 I dont think so no. I dont think theres an exact closed form for it.
did my first integration bee today and dominated cuz i binge watched all 60 vids in ur advanced playlist lol thanks
@@abdulllllahhh OMG AINT NO WAY!! DID U WON YOUR FIRST INTEGRATION BEE????
@ 5-0 in the finals (shoutout frullani) 💪💪
@@abdulllllahhh SHEEEEEEEEEESH!!! CONGRATS!!!
@@abdulllllahhh you mind sharing some integrals? 🥺
@@Silver-cu5up they were all lowkey relatively easy, like one of them was literally a simple kings rule so I instantly knew it was 𝛑/4, the other was a frulani with Arctan, another was 0 to 1 of (-logx)^-1/2 which just becomes Γ(1/2), another one evaluated to ζ(2) but I can’t remember what the integral was, 5th one was actually hard 0 to 1 of (loglog1/x)/(1+x)². Idk how they expected us to evaluate it, but I recognized the integral from Maths505 so I had a general idea of what to do. u = log1/x. Then take I(α) as 0 to inf (x^α-1)(e^-x)/(1+e^-x)² and notice that I = I’(1). Then use geometric series and rearrange to see that I(α) = Γ(α)η(α-1), differentiate and plug in 1 to get I = 1/2 log (𝛑/2e^γ) where γ is the euler mascharoni constant. Really wide variety of difficulty with these integrals lol
literally just became obsessed with ur channel and im already early lets gooo
oh shizz xD thanks and welcome!!
OLD UPDATE: I JUST FOUND A BETTER MOTIVATIONAL WAY of SOLVING THIS!! I'll make another video on this integral soon! It's similar method to the one in the video, but it's a more motivating and easier to remember approach!!! RECENT UPDATE: Here's the video: th-cam.com/video/UVKyiXnBo-I/w-d-xo.html
Timing myself: 1st Attempt: (2 minutes, 12.72 seconds) = 2:12.72 2nd Attempt (after knowing the process): 1:32.08 3rd Attempt (knowing the process better): 55.60 4th Attempt (memorized some answers of the process): 38.24 sec. Now if I have wait long, i should be slow again forgetting some answers in the process. Then remember it back and repeat until I fully memorized the shortcut to this integral. **SPOLIER: ** I'll end up remembering that: integral of sqrt(x+sqrt(x)) dx from 0 to 1 = 4sqrt(2)/3 - 1/8 [integral of cosh(2x)-1 dx from 0 to arccosh(3)].