An Interesting Exponential Equation | Problem 490
ฝัง
- เผยแพร่เมื่อ 7 ก.พ. 2025
- ▶ Greetings, everyone! Welcome to @aplusbi 🧡🤩💗
This channel is dedicated to the fascinating realm of Complex Numbers. I trust you'll find the content I'm about to share quite enjoyable. My initial plan is to kick things off with informative lectures on Complex Numbers, followed by a diverse range of problem-solving videos.
#complexnumbers #aplusbi #jeeadvanced #jee #complexanalysis #complex #jeemains
via @TH-cam @Apple @Desmos @GoogleDocs @canva @NotabilityApp @geogebra
FIRST PROBLEM: • Simplifying An Express... ❤️❤️❤️
Old But Gold: • Solving for a Complex ...
🤩🤩🤩 A Very Interesting Exponential Formula: zᵃ⁺ᵇⁱ = c+di: • A Very Interesting Exp...
🤩🤩🤩 An Interesting Locus Problem | Problem 399: • An Interesting Locus P...
❤️ ❤️ ❤️ My Amazon Store: www.amazon.com...
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you. ❤️ ❤️ ❤️
Recently updated to display "Books to Prepare for Math Olympiads" Check it out!!!
❤️ An Exponent That Doubles | Problem 353: • An Exponent That Doubl...
🤩 Playlist For Lecture videos: • Lecture Videos
🤩 Don't forget to SUBSCRIBE, hit that NOTIFICATION bell and stay tuned for upcoming videos!!!
▶ The world of Complex Numbers is truly captivating, and I hope you share the same enthusiasm! Come along with me as we embark on this exploration of Complex Numbers. Feel free to share your thoughts on the channel and the videos at any time.
▶ MY CHANNELS
Main channel: / @sybermath
Shorts channel: / @shortsofsyber
This channel: / @aplusbi
Future channels: TBD
▶ Twitter: x.com/SyberMath
▶ EQUIPMENT and SOFTWARE
Camera: none
Microphone: Blue Yeti USB Microphone
Device: iPad and apple pencil
Apps and Web Tools: Notability, Google Docs, Canva, Desmos
LINKS
en.wikipedia.o...
/ @sybermath
/ @shortsofsyber
#complexnumbers #aplusbi #jeeadvanced #jee #complexanalysis #complex #jeemains
via @TH-cam @Apple @Desmos @GoogleDocs @canva @NotabilityApp @geogebra
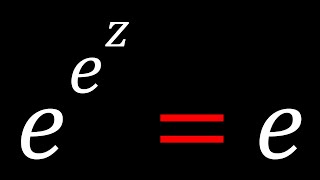








Cool!
Nice! From all of your channels aplusbi is my favorite😊😊💯💯💯💥💥💥💥💥💪💪💪💪
Thanks 🔥
I wonder if it is allowed to multiply both exponents by 1/n , then try to find solutions for m/n
Why not? Check the comment from @Don-Ensley
problem
( 1 + i ) ᵐ = ( 1 - i ) ⁿ
First obvious solution is m = n = 0.
Given n 0, raise to the power 1/n on both sides.
( 1 + i ) ᵐᐟⁿ= ( 1 - i )
Take natural logarithms on both sides. Bring down exponents.
(m/n) ln (1+i) = ln( 1-i)
(m/n) π(1/4 +2 k) = -π (1/4+2p)
(m/n) (1 +8 k) = - (1+8p)
(m/n) (8 k + 1) = - (8p + 1)
(8 k + 1) m = - (8p + 1) n
(m/n) = - (8p + 1) / (8 k + 1)
k, p ∈ ℤ
answer
(m,n) ∈ { (0,0), (- (8p + 1), (8 k + 1)),
(k, p ∈ ℤ ) }
Here's a geometric version. Think of 1+i and 1-i as hands on the clock, pointing at +/- 45 deg.
One 'tick' is one power increase - a movement of +/- 45 deg, with a magnitude increase of (^sqrt2.)
When do the 2 hands meat? 0 ticks at 0 deg, then 4 ticks at 180, 8 when they pass ea other again at 0 deg, etc. m = n = 4k, k >= 0.
Wow, that’s awesome! 🤩
e^(-i*k*pi)=e(i*k*pi) when n is even
k is even :)
m=n=4k I believe. I'll check with the video answer in a minute.
The first equality is because the base of both sides has modulus sqrt2, and therefore the end result of each side has modulus 2^(m/2)=2^(n/2).
Once we know m=n, we have a limited search space, and we only need to consider direction. The bases of the two sides have angles +/- pi/4, rotating this by pi/4 with each power, and the two nth powers meet up exactly when n is a multiple of 4.
Ok I did not look for complex solutions, that's on me. 😂
To be fair, there are weird considerations about branches and definitions for nonreal base and noninterger exponents. 😅
😄