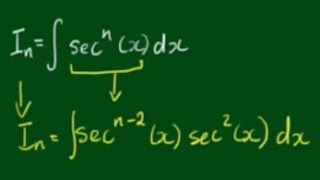
Reduction Formula for Integral of ∫csc^n(x)dx

In this video, we derive a formula for the integration of the powers of cosecant of x [csc(x)].
Our approach is to write the integrand csc^n(x) as csc^(n-2)(x)*csc^2(x), and now we have a multiple of 2 parts, and therefore we can use integration by parts to derive the reduction formula with.
u = csc^(n-2)(x)
and
dv/dx = csc^2(x)
Then we apply integration by parts and work our way to the solution.
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
I would really appreciate any small donation which will help me to help more math students of the world. Please donate here: paypal.me/Mast...
Follow me on Twitter! MasterWuMath

[@samuelsaldana1641]

Man you are a freakin hero, thankyou so much, please dont stop doing this kind of videos, im mexican and you just saved me

[@stasiawright377]

Thank you very much. Your video helped me a lot.

[@fentahunalex2781]

This is very good. Keep it up!

[@donaldmaviza4655]

please keep doing what you're doing.....the tutorials really help😥

[@mountainchicken766]

It was helpful for my work. Thx

[@cristhianvelandria9697]

You are they best

[@mathnesium8980]

Thank you teacher! Great video 😊

[@MasterWuMathematics]

You're very welcome!

[@innocenttwahirwa7761]

This method is very helpful, if possible give me reduction formula of tangent function with order n

[@HarshaVardhan-rr8uh]

Wow it was asked in my today's exam and I did it right!!!!

[@pianoplayer123able]

I also just did it right. No exam though.

[@WendsonBeyene-el8lx]

Ethiopia