Fundamental Theorem of line integrals
ฝัง
- เผยแพร่เมื่อ 7 ก.พ. 2025
- In this video, I present the fundamental theorem for line integrals, which basically says that if a vector field ha antiderivative, then the line integral is very easy to calculate. This illustrates why conservative vector fields are so important! I also provide a proof of the FTC, which uses... the chen lu!!! Finally, I show why we need the condition P_y = Q_x to check if a vector field is conservative. Enjoy!








This man's happiness when he does maths just makes me love maths even more
So clear! And so short! I never thought that this could be explained so convincing in so little time together with an example.
Quite possibly the best video I've seen on the topic, thank you!
Wow, thanks!
As you say “Thank you for coming”, just want to say “Thank you for teaching”!
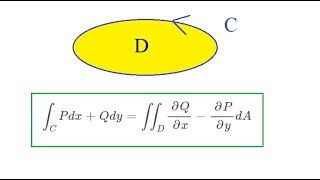
Important note: this only applies to conservative fields. In non conservative fields the line integral depends on the path.
That theorem is very interesting and very useful! Thanks for making this video!
at 6:45, dr peyam saying "arrrrgh but this- this is g(y)" is my new favorite thing in the world
Dr. Peyam, hope you are doing well! Thank you for all the wonderful content 😊.
OMG thank you alot now I understand it!!
could another reason the P_y Q_x test work be the fact that you can also find out if a vector field is conservative by the curl being 0 and the determinant definition of the curl would give you both of those in a term like (Q_x - P_x)k where k is the z-axis unit vector, meaning the term would have to go to zero?
You should do a video proving the equality of crossed partial derivatives!
very good guy, congratulations, your channel is awesome
I have a question , why u didn't after adding at the end in example ignored 2, Does that mean we have to add fx and fy always
Kyle from Nelk teaches Calc 3
Why does Clairaut's theorem (also known as: Swchartz theorem) can be applied when F is conservative?
It can always be applied, not just when F is conservative
It's dependant of the integration of parametrics functions of blackpenredpen ? If it's the case it's genial ! Thanks.
Those are two slightly different things, unfortunately! Here I show the line integral of a vector field is independent of parametrization. In bprp’s videos he shows a curve is independent of parametrization!
@@drpeyam Thank you very much fort your complete answer.
Why when taking a second derivative the order (dxdy dydx) doesn't matter?
They don't teach you that in physics 😿
It's something you learn in Calculus 3 (not Physics I believe) called Clairaut's Theorem. In this theorem you can see that those two derivatives are the exact same thing.
Experiment with derivatives mixing up the order, you’ll see it does not matter. As to why, well it’s analogious to multiplying. That is, 10.2.5 = 10.5.2 etc
thank you so much!
It was on 420 likes so I didnt want to like but had to give this a like now its 421 😂🤘🤘
Quixote is a character in a foreign book.
I think he said "quixotic" - which seems to be derived from the character, though :)
im struggling with this stuff
your smile makes me feel bad because i dont get it :(
:(
Check out my vector calculus playlist maybe?
And this: sites.uci.edu/ptabrizi/math2ewi20/
@@drpeyam I'll check it out when I finish this class. My final is Friday yikes.
@@drpeyam I have a 57% so far lmao I need like a 65% to pass the class with a c
Well, i'm sorry dr. Pi but you really had to explain that the first "check" (Schwarz Theorem) is a necessary but not sufficient condition for that to be an exact equation.
"Luckily" enough, that f(x,y) is differentiable and determined for every real x,y :)