
- 4
- 20 419
Eric Yu
เข้าร่วมเมื่อ 20 ส.ค. 2021
วีดีโอ
Geometric Intuition for the Computation of Dot Products, Cross Products, and Determinants #SoME3
มุมมอง 4.1Kปีที่แล้ว
A look at the connection between the geometry of the dot product, cross product, and determinant, and their methods of computation. 0:00 Dot Product: law of cosines 2:03 Cross Product: projection to a plane 5:09 Determinant: permutation of elements
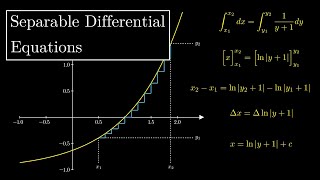
Intuition for Separable Differential Equations #SoME2
มุมมอง 15K2 ปีที่แล้ว
A visual approach to solving separable differential equations from the perspective of a first year calculus student. Let me know your thoughts in the comments. I'll put any corrections here in the description.
A Look Back at the Fundamentals of Arithmetic
มุมมอง 1.2K3 ปีที่แล้ว
www.3blue1brown.com/blog/some1 0:00 Introduction 0:30 Commutative Property of Addition 0:56 Associative Property of Addition 1:35 Subtraction 2:35 Multiplication 2:52 Commutative Property of Multiplication 4:10 Associative Property of Multiplication 4:33 Distributive Property of Multiplication over Addition 5:22 Division 6:32 Adding Fractions 7:25 Multiplying Fractions 8:12 Conclusion



Not gonna lie that's one of the best videos about linear algebra I saw
Brilliant!
We want more videos 🎉🎉
Thats some very helpfull intuition but i still have a problem with it . At minute 3:25 vector a and vector b are conviniently placed in such a way that they both have the same angle to the x/y plane. Needles to say ,most of the time thats not the case. So is there any other way to understand this intuition when the angles between the x/y plane and the a/b vectors differ ?
AxB is orthogonal to both A and B.
The confusing part is the projection of the second determinant a3*b1-b3*a1 it's not visually clear to me (that's the one in the middle of the 3 determinants). The relation between vector AXB and the axis where it is projected(this is the Y-axis) should be equivalent to the relationship between the AB plane and its projection(which is on the ZX plane) that is because the angles should be the same but I can't see that the angles are the same, what is the angle between AB plane and ZX-axis? Then the relation between the Y-Axis and ZX-plane should be equivalent to the relationship between Vector AXB and AB plane I think. Generally, I think this part should be better explained. Otherwise really great and useful video.
Thank you!
Great video
@yerictetris Is there any way to contact you?
Very nice video with crystal clear explanation
I still do not feel like I grasp the justification of extending the cosine to higher dimensions
Cross product is an ill-defined notion. I'd rather gave geometric intuition on wedge product, bivectors and stuff.
You’re a pro with Manim!
great video! Too many books just rather let me memorise the formula instead of understand the derivation, u save my life.
Awesome intuition!!
Great Video ! One remark I had while watching the video is that having the 3D plot constantly rotation was an issue for me, since I wanted to fully explore the details of the plot while you were speaking. Since the plot was always rotating, it was very hard to actually be able to get those details (I know that the plot was representing a simple concept, but I like to be able to really get any last details about something like this. It might have been some sort of approximation of the distances and such. They weren't necessary to understand, but it might be for others) otherwise the tone of the voice was awesome. You might want to give it a bit more life with more intonations, but I really enjoyed this video. The topic was awesome too. I learned dot and cross product at school, but never really got any answer as to why they behaved like this. The determinant of a matrix was also complete mystery for me. I always assumed it was a completely algebraic thing that someone found while trying to inverse matrices, but seeing that they have an actual geometrical reason + a "quick" formula to get any n-sized square matrices determinant was also great ! This video is very good and I wish you best of luck ! Please continue to find other "small" topics like this where you can make people eyes wide open :D
Third. Small intuition.
Second. Medium intuition.
First. Huge intuition.
Your video reminded us what a lot of people skip over, that maths is intended to make sense, whether in our physical world or derived from the more abstract worlds that we have earlier explored... Everything is just logic in this world of mathematics, and if something is not making sense, then we must be requiring some basic concepts that were skimmed over earlier in our journey
Thank you so much! This is exactly what I was looking for and struggling with articulating for the past 2 days.
Thank you!
Maybe if someone don't understand why you "can" divide and multiply by differentials, try to do the rigorous integral, in the sense that that in the "x" side, you are computing a Riemann's integral, and in the "y" side, you are computing a Stieltjes' integral.
very clean animation, nice
What a brilliant video, 3:28 was a particularly eye-opening moment for me!
how did you animate this?
Manim?
Engineers rewriting entire textbooks when a mathematician tells them that you can't treat Leibnitz's notation as a fraction:
As a physicist, derivatives are fractions, so it's already justified
As a physicist, derivatives aren't always fractions.
Wouldn’t the constant of ln|y+1| cancel with the constant of x?
No because they aren't the same constants. Some arbitrary constant c1 plus some other arbitrary constant c2 will result in another arbitrary constant c
Nice explanation!
I like what you're trying to do, and i like the animations and all. But the sound is so bad i had to stop after about half, please dont take this the wrong way. Just improve the sound. Content 10/10. Narration and context 4/10. Sound quality 2/10.
For sound, my score is 8/10 (points off for a bit of reverb). Is your sound output functioning well on your machine?
@@BlueSoulTiger and yeah I'm not having any sound problems on other videos or in games etc
Very Interesting and Informative. Very well explained! Great Job!
Nice editing skills, I'd love to see more content!
Very cool video. Definitely keeping this in a playlist for later reference.
this is very well done!
keep up the good work
Super well done, great animation and crystal clear explanations. Very helpful even when you've done a good amout of calculus :)
Think of dy as (dy/dx)dx then it's like u-sub
Eh. What you're referring to is a product of implicit differentiation.
yeah generally with this i like to not split the dydx and then integrate both sides dx so you get dy/dx=g(x)/f(y) -> f(y)dy/dx=g(x) -> int f(y)dy/dx dy = int g(x) dx -> int f(y) dy = int g(x) dx which idk feels ike it doesnt really run into any issues
Amazing video, for the integral step my teacher showed me that actually you don't need to split the derivative, for f'(x) = f(x) + 1 - > f'(x)/(f(x) +1) = 1 we can simply integrate both sides in respect to x and because of the chain rule, integrating the LHS in respect to x is the same as ∫1/(y+1) dy (the dy/dx is the 'conversion factor' transforming the dx into dy - like when integrating with substitution) its hard to explain clearly in a comment but hopefully that makes sense ∫1/(f(x)+1) * (dy/dx) dx => ∫1/(y+1) dy
This is what my ODEs professor did as well.
I have been studying calculus on my own for some time now and while doing so I have always yearned to intuitively understand all of the mechanics behind it. With regards to these separable differential equations, treating the derivative like a fraction was never unintuitive for me because I've always understood it to actually be a ratio that could be manipulated. But integrating both sides of these equations with respect to different variables had always felt handwavy to me even when I learned of the more rigorous abstract justifications. This video finally clarified why this method works, and I've got to say thank you for that. Geometry will always be the backbone of math for me. You are truly awesome!
Great video. I'm a tutor and this will help me explain these fundamentals in a logical way to my students
I would tend to think that the easiest way of justifying this manipulation would be to go from f'(x) = f(x) + 1 verified for all x in an interval I, assume f does not equal -1 anywhere on I, and divide by f(x) + 1. You then have, for all x in I, f'(x)/(f(x)+1) = 1. The left-hand side is the derivative at x of x -> ln|f(x)+1| and the right-hand side is the derivative at x of the identity. The equality between derivatives is true over an interval I, therefore the functions are equal modulus some additive constant. You thus end up with the same result. Really, all separable differential equations (at least ODEs) could be solved in this way, without ever writing dx or dy anywhere. The separation of infinitesimal quantities makes sense for me only in the context of physics or engineering or chemistry, etc, because in those domains you actually very often handle infinitesimals. In maths, the least you can use these notations the better, in my opinion. They just confuse matters, and hide the fact that there is a perfectly clean way to do the same thing. Still, the video is really well written and animated! I hope my comment doesn't come across as too critical, because I could never critise what I can't do and I certainly couldn't make such a good video. Well done, and best luck for the #SoME2.
this is a really nice visual explanation with amazing animation. well done! :)
Great intuitive explanation. Some might still have qualms with the whole separating the dy and dx in leibniz notation (despite this being by far the most common way of teaching them), and I think there is also a nice way to understand separable diff eqs without that 'hand-wavy' shorthand. When solving a separable differential equation, the actual integration you're performing is (by necessity) with respect to the same variable on both sides. In reality, you're actually performing the reverse of the chain rule. However, the Leibniz notation manipulation works and can be intuitive, I just think seeing what's really happening is good for a student to see at least once. Here is an example of what's really happening behind the scenes: Let's say you have a separable diff eq of the form: g(y)y′=f(x) (this is clearly separable, and any separable diff eq can be manipulated to this, this specific form is purely to make things easier for this example). You can imagine going in the other direction if you differentiated some known solution G(y)=F(x) w.r.t. x, that extra y' would pop out on the left due to the chain rule to leave you with g(y)y′=f(x). This is the opposite process of solving a separable ODE. So when solving these diff eqs, you're actually pulling some terms over to the side with the derivative and reversing the chain rule to handle with the different integration variables. Integrating f(x) will give you F(x), and integrating g(y)y' w.r.t. x is the same as integrating g(y) w.r.t. y. I would highly suggest messing around with the chain rule to convince yourself of that. I may not be the best explainer but when I discovered this I felt it gave me a better understanding of the actual math at play rather than a shorthand. Im sure people on google have better explanations than me, some random commenter if you want to look further. I hope you continue making videos and sharing the beauty of math! Creators (no matter the size) are the biggest modern driver of people understanding math is beautiful and not doing times tables. Keep up the great work!
Thanks for the feedback! I think that as a consequence of the fundamental theorem of calculus, there are often two different ways to approach problems like these: thinking in terms of slope, and thinking in terms of area. One example of this is the method of u-substitution for evaluating integrals. On one hand, it can be thought of as the chain rule in reverse, since setting u equal to the inside function can reveal the way in which the functions are composed. On the other hand, it could also be thought of as applying an area-preserving transformation to the integrand and adjusting the bounds accordingly. In the case of separable differential equations, I personally think that reasoning in terms of how each dx relates to each dy, and what that implies about the function as a whole, feels more motivated and natural, and isn't necessarily less rigorous, than trying to fit the equation to a form that we know works when going the other way.
@@yerictetris While it does feel more motivated and natural, but it is also certainly less rigorous. Those are terms that are coupled results from limits and can not, in general, be manipulated and integrated in this intuitive manner. The dy/dx notation is a single term, not an actual ratio. These 'Infinitesimal' terms are not just finite numbers and shouldn't be manipulated like this in all cases. However, most practices in engineering or physics will even recommend doing it this way, because it is easy, and it does make complete intuitive sense, especially when the focus is on practicality and not mathematical rigor. This is a use of 'nonstandard analysis' and can be extraordinarily useful. Both thoughts of U-substitution you mentioned are identical, the mentioned transformation is a correct representation of what is happening when you reverse the chain rule. Any visual interpretation can still be inferred from performing the chain rule in reverse. They're not separated interpretations they are accurate descriptions and two sides of the same coin. Splitting dy and dx, as said by you "seems completely unreasonable, and is a blatant abuse of notation." The point of my comment was to just point out that when putting pen to paper, the real reason that it works out is due to this process. It's not a different 'way to think about it', it is what is actually happening, and all visual interpretations are still a result of this process. Of course, just saying 'reverse the chain rule' is still not rigorous (which I think you were getting at), and further proving takes more knowledge of why exactly the chain rule works, and why the FTC works using limit definitions/real analysis, but it does at least improve the explanation so that it doesn't just abuse notation. Your geometric interpretation is certainly not at odds with what I am saying, it is an intuitive explanation of why what I am saying works. (And again to be clear I still solve separable diff eqs by splitting dy and dx, and would recommend pretty much anyone do so, I just think its good to know why you are able to do that) TLDR: You cant integrate w.r.t different variables, and splitting dy/dx is certainly not rigorous, my comment was to explain a little why it still works, and intuition is still a great thing.
@@ethandennis3803 I think I see what you mean. If dy and dx are separated so that they're on different sides of the equation, then taking the limit as dx approaches zero kind of loses meaning, since both sides will evaluate to zero. However, what if we did this: For a function y = f(x), define dy to equal f(x+dx) - f(x) given some arbitrary non-zero dx. We can now see that lim_dx-->0 (dy/dx) equals the derivative of the function by definition. Now we consider the equation dy/dx = y+1. We're not taking any limits yet, so for now, dx is just some arbitrary non-zero number, and dy/dx is just an approximation for the derivative. We can freely manipulate the equation to get dy/(y+1) = dx. Therefore, taking the sum of both sides, Σdy/(y+1) = Σdx from some point on the function to another point. Finally, we evaluate the limit as dx approaches zero of both sides: lim_dx-->0 [Σdy/(y+1)] = ∫dy/(y+1) lim_dx-->0 (Σdx) = ∫dx Therefore, ∫dy/(y+1) = ∫dx, and we've done it. TLDR: Delay taking any limits until after the summation. Let me know what you think.
@@yerictetris So a problem here is you are still doing the discrete version of the old problem, but I actually think it serves as a useful explanation of why, and what happens if you 'take the sum of both sides.' You can not actually just take a sum with 2 different variables and bounds, as that is performing an inconsistent operation on both sides. So you are taking a statement that you have set by definition to be equal: dy/(y+1) = dx. Those sides are the exact same thing, and in general, as long as you perform the *exact same operation on both of them* , they will be the same. Adding up dys on one side and adding up dxs on the other side is not the same operation, but *the way in which they are coupled* makes it work out in the case of the integral. When taking the sum, you need to think about what you're adding and what the bounds are, as well as what the finite change you are iterating is. With an inconsistent variable bound, and an inconsistent Δ, it just doesn't make any sense. Summing over 2 different variables is just simply not the same operation. Taking the sum of your dy/(y+1) = dx equation (I'm choosing with respect to x) would in reality give some Σ(dy/(y+1))Δx = ΣdxΔx in order to be performing a consistent operation. Note that in the integral version it also doesn't actually make sense to integrate over some existing dx or dy, but its harder to tell when the numbers are an abstract concept of 'infinitesimal' numbers. So what if we try to use the existing Δx as our step in our sum? Well lets walk through that: Your finite equation is Δy/Δx = y+1, this can be rearranged to be (Δy/Δx)*(1/y+1) = 1. We can now take the sum of both sides w.r.t. x and with a consistent Δx. This gives us Σ(Δy/Δx)*(1/y+1)*Δx = Σ1*Δx. The right side works out easily, but the left side does not. Sure this can kind of be simplified to your form of ΣΔy/(y+1) = ΣΔx, however, the bounds of both sides are still w.r.t. to iterating x according to Δx. Also remember that y, as well as Δy/Δx are a function of x. I imagine when you wrote out your comment you pictured the left sum to have some sort of y bound and iterate with Δy. How can we reconcile this sum then? Well as far as I know (am inexperienced with discrete math, and have not thought super far into this, so there definitely could be a way), we can't really. But the integral version is super easy - it just becomes ∫f'(x)*(1/(f(x)+1))dx. This can clearly be solved just by reversing the chain rule. (or as you may be taught in first-year calc, u = f(x), du = f'(x)dx, should be simple from there). This comes full circle to how what you really are doing is reversing the chain rule. However, again I cant stress enough, the intuition you have is fantastic, and if you are going into most math-heavy fields, it works great. I am in the field of dynamics, and I think how you think about most things. Especially in any field of physics, dx and dy are used super commonly, and this shorthand is almost always a good intuition. Also please don't take me as this being rude or shooting down your arguments. I just think the mathematical background is interesting and can be important to see behind the common shorthand and intuition. (This was also just off the dome, and am not saying I'm 1000% right about everything, but that was the flaw I saw in your argument. What I can say confidently is that many people much smarter than me would agree that splitting dy and dx is not rigorous, though can be intuitively useful)
@@ethandennis3803 Your perspective here of having the same ∆x on both sides made more sense to me, and integration w.r.t. the same change of variable is what makes the LHS and RHS coupling "obvious". The separable variable method seems to work as well as it does IMO (regardless of how you play around with notation), is probably because there isn't a self-dependency between the coupling of the two variables in the first place. An equation like dy/dx = x+y is not solvable using this method because the derivative is dependent on both the independent and dependent variable. This is where the abuse of notation becomes obvious - taking the dx denominator onto the RHS as numerator and then trying to integrate is problematic because you are now trying to deal with the y•dx which appears as a composite function to the Integral. 1 solution to this is to convert the composite function into a single variable using u=x+y and then using the variable-separable method on the single variable so that the self-dependency is resolved into indenpendency.
Nice vid! I'm curious how you animated it
For this one I just used Powerpoint.
Fantastic! You found just the right visual for each of the concepts. Completely agree about learning the fundamentals well and having an intuition for the symbols being used. I had a similar topic for my submission, I would love to hear your opinion on it :)
Totally agree! Especially for fractions. Great job!