วีดีโอ

An easier solution is to make the limits variable using the substitution x-t=k and then use the lebinitz formula and use maxima minima concept.

Beautifully explained at every point..❤❤❤.

Sir eek pyqs hai x+2y+3z=42 , jee mains 2024 ka 1 Feb , plzz wo karwa dijiye , uska net par soln kafi lengthy hai

Sir ek question joh topic aap batate hai waisa sa hii ek question extra leke aaya kariye and usko as home work de Diya kariye

Pnc n probability plz

Sir ek saath zyada ques laiye plz

the question framing is crazy

phle maine socha tha ki Identity bna ke fn nikl lu then solve karu but nhi hua

what an amazing soln

Exam me aise hi q maths ke percentile increase karte

Very clever approach in solving inequality Thanks sir

sir please pnc ke questions

can be more quick 2/3 of rectangle

Nice

Thank you sir really helpful ❤

Sir iske ke andar ek aur idea hain, [x²] ka integration from 0 to some constant lene mein √2,√3 karke saare radicals aayenge and tthey cant be eliminated so alpha ki value iss format mein 2 hi hogi.

i am still worthy😅..

Amazing video sir loved it ❤️

Stuck in remembering the formula 😤

The question is unnecessarily lengthy, combining concepts from 3D geometry, integration, and the greatest integer function. It feels like an exercise in pure labor rather than testing genuine knowledge. The amount of time required to solve it is excessive, and the level of conceptual understanding needed is minimal. The question seems to prioritize complexity and calculation over testing deep understanding or problem-solving skills for jee But once again your teaching is excellent My 🫡 salutes to ur knowledge and inspiration and teaching sir

😃

A fster method would be to notice that g(x).ln part is an even function Integrating an even function(definite integration) give odd function Thus f(x) is odd and integrate f(x) gives 0

Ek do hw bhi dediya kro sir

What an amazing method of solving sir thanks

Thank you sir for discussing best question of jm

WHAT about x=0 ? It is also a root

Ye question olympiad se utha hai

Thanks sir, could you take up this one question of quadratic equations from SEPTEMBER 21ST 2021 jee mains ( the number of pairs (a,b) ........ )

Sir please evaluate this question Let f : ( − ∞ , ∞ ) − { 0 } → R f : ( − ∞ , ∞ ) − { 0 } → R be a differentiable function such that f ′ ( 1 ) = lim a → ∞ a ^2 f ( 1 /a ) . Then lim a → ∞ a ( a + 1 )/2 tan^− 1 ( 1/a ) + a^2 − 2 log e a is equal to

Cartesian graph par plot kar ke kiya tha yeh question

Sir please matrices + pnc Wale ques pe video plzzzz 🙏🙏

Complex no. Meets geometry meets sets meets inequality 🎉😂

👍

First time i did this question in definite integration class test i just assumed that f will be odd without working that part out luckily i was saved. 😅

Good question

👌🏻

Love your consistency. Way to go❤

Ez in 2 mins

Those 100k from 2.6k will come soon💫

Super solution. I am a senior JEE Maths faculty.

Sir ...why did you conclude that modulus will open according to value of x?

Fucking Good problem!💀

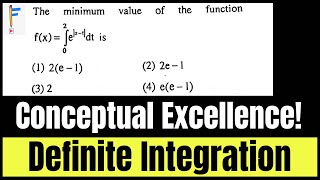
sir, f(x)= 0 to 2 int e^|x-t| dt, isme agar x=t put kare, f(t)= 0 to 2 e^0dt =2 hua minimum?

amazing

Absolutely helpful ...👍

Respected Sir can we do like this When the value of x is greater than 2, the function behaves in such a way that it equals a shifted version of itself. As x increases, the curve continues to rise above a certain point, indicating that the curve has a maximum value and then starts to fall as you move further to the right of x equals 2. On the other hand, when x is less than 0, the curve starts from negative infinity and rises up to a specific point. This shows that the curve has maximum values at both ends, specifically at x equals 0 and x equals 2. Therefore, these points are maximum points, not minimum ones. Now, between the range of 0 < x < t where t is less than 2, there should be one point where the two parts of the function intersect. The function takes two forms: one decreases and the other increases, and they can only be equal when x and t are both equal to 1. Taking into account the symmetry of the function, you would integrate a certain expression, and the result of that integration So it is twice of integration between zero and 2 of e power 1 -t with respect to t Sir this gives the final answer as twice the difference between the constant e and 1.

For minima always symmetry is obtained so make graph symmetrical about x = 1 and double the integral of e^x from 0 to 1 oral answer 2(e-1)

We really need such channels which can provide Good solutions to pyqs .Thank you so much sir.

keep it up 😊

What a beauty ❤️❤️