
- 10
- 25 636
Vantage Admissions
เข้าร่วมเมื่อ 14 พ.ค. 2023
Vantage Admissions are a specialist admissions consultancy providing support for students taking mathematics entrance exams and interviews for elite British universities, such as STEP, the MAT, and the TMUA. From detailed past paper solutions to mocks marked by real examiners and taught courses covering the intricate ideas used in these exams, our comprehensive programmes have helped many students to receive admission to their dream university.
To learn more, visit www.vantageadmissions.co.uk.
To learn more, visit www.vantageadmissions.co.uk.
STEP 2 2022 Question 1 Solution - Integration by Parts - Vantage Admissions
Rowan Wright from Vantage Admissions presents the solution to Question 1 from the STEP 2 2022 paper, demonstrating the clever use of integration by parts to solve challenging integrals.
At Vantage Admissions, we offer a comprehensive STEP preparation programme. The programme is authored and delivered in its entirety by Rowan, who is a past STEP examiner with over 7 years' experience as a specialist tutor. To find out more, visit our website and book a free consultation today!
STEP Programme: www.vantageadmissions.co.uk/course/sixth-term-examination-paper
STEP Resources: www.vantageadmissions.co.uk/resource/step-resources
Knowledge Base: www.vantageadmissions.co.uk/knowledge-base
© Vantage Admissions Ltd 2025
00:00-00:19 Introduction
00:19-06:44 Part (i)
06:44-11:42 Part (ii)
11:42-16:22 Part (iii)(a)
16:22-19:35 Part (iii)(b)
19:35-23:21 Part (iii)(c)
At Vantage Admissions, we offer a comprehensive STEP preparation programme. The programme is authored and delivered in its entirety by Rowan, who is a past STEP examiner with over 7 years' experience as a specialist tutor. To find out more, visit our website and book a free consultation today!
STEP Programme: www.vantageadmissions.co.uk/course/sixth-term-examination-paper
STEP Resources: www.vantageadmissions.co.uk/resource/step-resources
Knowledge Base: www.vantageadmissions.co.uk/knowledge-base
© Vantage Admissions Ltd 2025
00:00-00:19 Introduction
00:19-06:44 Part (i)
06:44-11:42 Part (ii)
11:42-16:22 Part (iii)(a)
16:22-19:35 Part (iii)(b)
19:35-23:21 Part (iii)(c)
มุมมอง: 185
วีดีโอ
Oxford Maths Interview Question - Vantage Admissions
มุมมอง 6K2 หลายเดือนก่อน
In this video, Oxbridge admissions expert Rowan explains the solution to a recent Oxford Maths interview question. Make sure you practise explaining your reasoning aloud if you attempt the question before watching the solution! At Vantage Admissions, we support students preparing for interviews in mathematical subjects, including Mathematics, Computer Science, Economics, Physics, and Engineerin...
Cambridge Computer Science Interview Question - Vantage Admissions
มุมมอง 8922 หลายเดือนก่อน
Oxbridge admissions specialist Rowan Wright presents an authentic problem asked in Cambridge Computer Science interviews. Rowan works through the solution step by step, explaining how you might generate similar ideas. At Vantage Admissions, we support students preparing for interviews in mathematical subjects, including Mathematics, Computer Science, Economics, Physics, and Engineering. We offe...
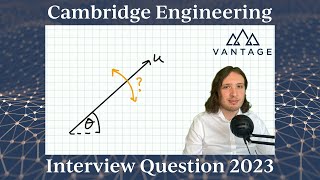
Cambridge Engineering Interview Question - Vantage Admissions
มุมมอง 1.6K2 หลายเดือนก่อน
In this video, Rowan works through the solution to an Engineering interview question asked recently at Cambridge. ✍️ This question was asked to Engineering applicants, but is also representative of the questions asked to Physics (Physical Natural Science) applicants. Rowan demonstrates the all-important 'thinking out loud' required to excel in Oxbridge interviews. At Vantage Admissions, we supp...
Oxford Physics Interview Question - Vantage Admissions
มุมมอง 9562 หลายเดือนก่อน
What are Oxbridge applicants asked in a Physics interview? 🔭 In this video, Rowan presents a real interview question asked recently at Oxford, complete with the fully worked solution. Rowan is an experienced university interview coach, with over 7 years' experience in supporting students applying to top UK universities. This question is also applicable to Engineering applicants because they are...
Cambridge Maths Interview Question - Vantage Admissions
มุมมอง 4.9K2 หลายเดือนก่อน
In this video, Rowan expertly guides you through a Maths interview question asked recently at Cambridge. As specialist tutor and interview coach with over 7 years' experience, Rowan explains the thought process in detail and demonstrates the style of communication required in Oxbridge interviews. 📚 At Vantage Admissions, we support students preparing for interviews in mathematical subjects, inc...
Oxford Computer Science Interview Question - Vantage Admissions
มุมมอง 9182 หลายเดือนก่อน
Preparing for an Oxbridge interview in Computer Science? 🎓 In this video, Rowan Wright works through the solution to a real interview question, asked recently at Oxford. Rowan breaks down the thought process step-by-step, and demonstrates how to communicate your ideas clearly. At Vantage Admissions, we support students preparing for interviews in mathematical subjects, including Mathematics, Co...
Cambridge Maths Interview Question - Vantage Admissions
มุมมอง 7Kปีที่แล้ว
This is a worked example of a interview question asked at Cambridge in a recent Mathematics interview. At Vantage Admissions, we offer a comprehensive preparation course for Oxbridge interview in Mathematics and Computer Science. It comprises over 11 hours of taught content, 150 original worksheet problems, and a question bank of 80 interview questions asked from 2019-2022. We are proud to offe...
TMUA 2022 Solutions - Paper 1 - Q11-20
มุมมอง 1.8Kปีที่แล้ว
For Q1-10, see th-cam.com/video/quwc5O9SOTE/w-d-xo.html At Vantage Admissions, we provide intensive preparation programmes for students preparing for MAT, TMUA, STEP, and Oxbridge interviews. This includes access to detailed past paper solutions in both video and booklet form. Since TMUA is being discontinued from 2024, it appears that Cambridge are not releasing worked solutions to TMUA 2022. ...
TMUA 2022 Solutions - Paper 1 - Q1-10
มุมมอง 1.4Kปีที่แล้ว
For Q11-20, see th-cam.com/video/Xe9qnNBt5cQ/w-d-xo.html At Vantage Admissions, we provide intensive preparation programmes for students preparing for MAT, TMUA, STEP, and Oxbridge interviews. This includes access to detailed past paper solutions in both video and booklet form. Since TMUA is being discontinued from 2024, it appears that Cambridge are not releasing worked solutions to TMUA 2022....








Easiest JEE mains definite integral
this genuinely wasn't difficult. he walked through it elegantly and it seems like you should be able to as well if you've got a decent handle on combinatorics/dp
1 (iii) (b) IBP not necessary since ∫e^x[f(x) + f'(x)]dx = e^xf(x) + c
You are certainly correct, although morally this is really equivalent to IBP since that result come from the product rule and integration by parts is simply a formalisation of the 'reverse product rule'. Sometimes showing more steps can be a good idea as it means we are more certain to receive method marks and, even if the shortcut is mathematically correct, it can also reduce the risk of unfriendly marking errors! - Rowan
Interesting, but does not seem likely to encounter the general case.
Wow
I am a retired Maths teacher. I was extremely impressed with the quality of the explanation here. Well done!
Thank you for your very kind comment -- clarity is certainly the goal! - Rowan
Top tier video 👌 An alternative would be finding the max height and range of the projectile (by making angle 0 and 45 degrees). Then you have the coordinates of your turning point (0, max height) and your roots for the parabola (range) And you can "un-complete" the square to find your equation
For the last part, could you just do 2 + an infinite series 2/4, 2/16 etc. which gives 2 + (1/2)/(1-1/4) = 2+ 2/3 = 8/3
f(x)=(π/2)*sgn(x+1)+(π/4)
You can also view this as a random walk, and use the optional stopping theorem.
yeah but for university admissions, unlikely to expect students to know about this stuff.
Do you provide a course for STEP / MAT? If so how much is it
Yes! You can read about our MAT course here www.vantageadmissions.co.uk/course/mathematics-admissions-test . Our STEP course is slightly changing for 2025 (lessons will be pre-recorded rather than live to afford better flexibility, with the weekly live lessons then replaced with office hours) but the basic format and price should be the same when our STEP 2025 programme launches in early December www.vantageadmissions.co.uk/course/sixth-term-examination-paper . I am happy to discuss further in a free consultation which can be booked on our website. - Rowan
i would cry if i got this question
nice question not too badd
cld u do an econ oxbridge q pls
Yes - stay tuned!
The first part is really easy but I am to lazy to skip ahead to see how hard the second part is
There are clearly lots of integration wizards in the comments who know all about special integration tricks including the 'King's rule'; that's just a name for one of the tricks used in this video. It's important to remember that, in an interview, they will want to understand your thought process. If you do state that you are using the 'King's rule' rather than sniffing out the correct approach in the more hands-on way used in the video, it's vital that you're prepared to justify *graphically* why this property holds, as they are very likely to press you on it. The point is that f(a+b-x) is the reflection of y=f(x) in the line x = (a+b)/2, which is the midpoint of an integration interval [a,b]. That means the integral from a to b of f(x) is the same as the integral from a to b of f(a+b-x), because the latter integral is giving the mirror image of the same area (which therefore has exactly the same size). - Rowan
Might be worth it to know the FTC proof as well (as long as you can explain pretty well where FTC comes from).
@@-taehyun Whats the FTC proof please teach me bro
@@宻 Fundamental theorem of Calculus i.e integral of f(x) from a to b is F(b) - F(a) where F'(x) = f(x). if you look at f(a+b-x) and use a substitution u = a+b-x you will find that by swapping limits and evaluating by our previous rule (FTC) that you get F(b)-F(a), the same result as evaluating the integral of f(x). Bit hard to explain through a youtube comment i'll latex up a version and send it through later.
How do you know someone is preparing for IIT? They'll tell you
we can solve this very easilly thanks to the King propriety
Thanks so much for these videos Mr Wright! These videos are very helpful, could you please upload some more CS interview questions in the future. I'm sorry but I can't afford the course.
Certainly stay tuned for more! - Rowan
A nice example in which the usual integration techniques don't work. The power k does not have to be an integer. A similar integral ,for which the same reasoning could be applied is of the form ∫1/(1+ f[x]/f[x-a]), 0 < x < a .
You are of course completely correct that k needn't be an integer. I suspect this restriction was given so that trying k=1 first would be an accessible option; once seeing that this game worked for that case, students would then hopefully realise that the fact the power was 1 was not actually important and the argument worked more generally. - Rowan
Solved this one in my head, I= integral 1/(1+(sin(x)^k/cos(x)^k)) dx rewrite the integral as cos(x)^k/(cos(x)^k+sin(x)^k)), you see that you take the pi/2-x usub and you add it to the original integral, and you get 1. thus, for all k in the natural numbers, this integral is pi/4.
3:20 whoah!!! That's fire! 😂😂
aha saw it was king's rule straight away -- super useful. queen's rule and jack's rule i think are starting to be used now too, so good to learn those too
Yes, you're absolutely right -- any tricks which are ultimately based on graphical symmetries (for instance, the trigonometric identities we noted are equivalent to the fact that sine and cosine are each other's reflection in x = pi/4) are very important for these interviews and are well-worth getting used to! -Rowan
how do i learn those?
and are there any other niche rules like kings,queens, jacks rule i should know for interview preparation in general? many thaknks
@ kings rule, jacks rule, odd and even functions, principle of inclusion exclusion (then the derangement formula which can be derived from this) if i think of any more i will drop them in the comment. you can google to find out more about these
@@宻 As well as these sorts of tricks with names, as far as integration is concerned it's really important when preparing for maths interviews to build a rock-solid grasp on the more standard techniques. For example: think carefully about how you might spot a good substitution beyond simply substituting the 'complicated' bit of the function (examples: trigonometric substitutions motivated by trig identities, u =f(x) substitutions which proceed more smoothly since f'(x) is a factor in the integrand and then the f'(x)dx nicely becomes du); think about how you might spot a good application of integration by parts (LIATE is a useful acronym here, and you should also think about tricks like writing f(x) as 1 * f(x) to allow IBP, and 'partitioning powers' e.g. in x^2 e^x^2 letting one of the x's from x^2 go with e^{x^2} as dv/dx so that you can integrate it). Also, the trick used in this question where we essentially proved that the integral solved an equation, I = pi/2 - I, rather than 'solving' in the traditional sense, is used very broadly beyond just 'king's rule'. - Rowan
i despise q3. The 'expected' number of throws would be undefined, because you cant ensure that you definitely will get a choice.
When we talk about ‘expected’ in the sense of a random variable, we don’t mean in a deterministic sense of anticipating a precise value, but rather the definition as a sum given in the video. One way to think about it intuitively is the mean average result you would get if you repeated the experiment a very large number of times. There are indeed cases where this notion does not align well with intuition - e.g. some random variables may have an infinite expectation. See, for instance, the St. Petersburg Paradox. Luckily, in this case, the notion of expectation actually works very well!
Thank you mate 🙏🙏 I got a cambridge offer last month. Sadly I need a S,1 in step which ive never heard of, I'm assuming it's because I was borderline ability
Congratulations! Rest assured the S,1 offer will not have been because of any lack of interview performance on your part. Rather, several colleges seem to have decided to make S,1 offers across the board recently and especially this year. For instance, we had multiple students receive offers at Pembroke, all S,1. Traditionally S,1 offers were mostly made to reapplicants and almost always such students have still been admitted if receiving a 1,1. So, certainly aim for the S, but in all likelihood you will be accepted with 1s too. It’s not too late to join our STEP 2024 programme, so don’t hesitate to get in touch if you would like to hear more! - Rowan
The whole concept of entrance exams stinks. If your teaching skills are as good as you say, then you should be able to apply them to everyone, not just those who don't really need those skills because they are already ahead. Depends what your view of the end result for society is. Increased inequality, or increased equality? Clearly OxBridge is built on the former.
I don’t think Oxbridge has better ‘teaching’ but rather it kind of teaches/focuses on different things. The entrance exams are there to try to ensure that will work for the students given the time constraints
an oxbridge degree would be worthless if they lowered the standards to the point where the average joe could ace it. the courses are intense, and competitive, and as such they have to have a rigorous admissions system. if you don't like that then just don't apply.
Well, concepts cannot be explained if someone doesn't have the pre requisite knowledge about the concept. For example, you won't be able to explain what prime numbers are to someone who doesn't know maths. The high expectations are so that they get students who has already a lot of background knowledge and can therefore connect with the lecturer much more easily than someone getting a B or C for example. Uni content at those universities is very intense and if the lecturer has to explain everything, then it would take ages to finish the degree. Getting someone who is appropriate for a task isn't unfair, given the task could have done by any human being.
I havent watched the video yet but here are my solutions: 1) THrow two coins and do this if HH -> apple elif HT-> banana elif TH-> carrot else (TT) repeat this whole process again 2) Two find P(apple) i do 1/4 + 1/4*4 + 1/4*4*4 + ... this is a converging geometric series By using the formula of sum to infinity with a = 1/4 and r = 1/4 I get the sum as 1/3 which is perfect 3) Funnily enough the expected number of throws should be infinity. Not sure about rigorously proving this but however unlikely it is its possible you keep throwing tails forever. And because of how infinity breaks math as I know it i think this should mean multiplying infinity by very small fraction doesnt change the fact its infinity.
Question 14: The angles around the centre for each triangle should be 2π/3 not π/3. You still get the correct answer because sin(2π/3) = sin(π/3).
Thank you very much for spotting this - you are absolutely right that two of the three triangles have that centre angle mislabelled. This will be corrected in an annotation.