วีดีโอ

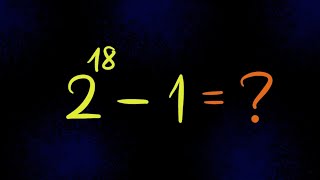
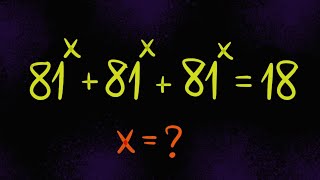
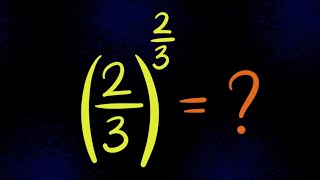
i know two powers to 30 so i know its 262144 - 1 = 262143

1/a +1/b = 1/11 11/a+11/b =1 * x/y+x/z=1 *y=z=2x • a = b = 22 a+b = 44 1/22+1/22 = 1/11 ✓

2^18-1=2^10*2^8-1 = 1024*256 - 1 = (1000+24)(250+6) -1 = 250000+6000+6000+144 -1 = 262143

this just reminds me how much "show your workings out" annoyed me age 10-17yo - "but that slows me down, why would you want 3 questions answered in the time I can give you 10 questions - even infuriated when asked "I can see some workings out, but how did you get from this step to this step?" "ughhh its obvious, why do I need to write, timesing by 15 is times 10 plus its half, dur 5 is 10/2 - I guess you want me to write out the long division rather than looking at the number and basically seeing it's half"- "you do?!?! wtf"

rezultat a+b=11] =[[ 4^7=7+4]=11]a^b=a+b

This only works if a and b are restricted to being integers for you to be able to say (2b+1) and (2a+1) must equal 1 and 17, or -17 and -1. I don't think that's ever specified anywhere.

a(sqrt(asqrt(a)))=2 a^2(asqrt(a))=4 a^6*a=16 a^7-16=0 Solution 1: a=2^(4/7) Solution 2: on the complex plane, n^7 has to be 16, or in other words, 7θ=2pi θ=2pi/7, r=2^(4/7); a=2^(4/7)(cos(2pi/7)+isin(2pi/7))

x = 10, y=8 10^2 - 8^2 100 - 64 = 36 : )

0,5lg15/lg5. NOTE. The other roots are complex numbers.

❤❤❤❤❤❤

,, log(2) " , log(2)x*log(2)x=9 , log(2)x=+/-V9 , log(2)x= 3 , -3 , 2^3=8 , x=8 , 2^(-3)=1/8 , x=1/8 , solu , x= 8 , 1/8 ,

1/8 and 8

El resultado final no es exacto, es una aproximación decimal acotada a tres dígitos. El resultado exacto tiene infinitas cifras decimales sin repetición periódica por lo que el resultado final es un número irracional

El procedimiento es muy extenso sin necesidad...

√2+√x=2. Solución: La solución es para x mayores que 0 → √x=2-√2→(√x)²=(2-√2)² →x=2²-2(2)(√2)+(√3)² x=4-4√2+3→x=7-4√2 o Resultado: x=1,3431

why not just use pascal's triangle?

Congratulations on being incredibly stupid! Clearly you should move the sqrt(2) to the other side.

wouldn't it be easier if you get sqrt(2) to the other side and you get sqrt(x)=2-sqrt(2)? now you square both sides and voila. x=6-4sqrt(2)

WRONG. WTF? x can not be greater than 2. Olympiad for kindergarten? Check your work before posting IDlOT.

First of all, it can't be an Olympiad question Second of all, you even solved it wrong qwq you literally introduced an extra root to the equation

Binomial expansion of the 10th power is painful; but expansion of (3 - 2√2)^5 took me less than 2 minutes with mental math as = (3^5 + 10 * 3^3 * 8 + 5 * 3* 64) - (5 * 3^4 * 2 + 10 * 3^2 * 16 + 128)√2 = (243 + 2160 + 960) - (810 + 1440 + 128)√2 = 3363 - 2378√2.

Squaring the thing twice, what can go wrong? Like, maybe, introducing extra roots that shouldn't be there? What's wrong with sqrt(x) = 2-sqrt(2) => x = (2-sqrt(2))^2 = 4 -2*2*sqrt(2)+2 = 6-4*sqrt(2)? (with the 6+4*sqrt(2) being totally wrong).

вообщето количество корней равно степени уравнения тоесть умножаем обе чести на левый делитель потом переносим всё в левую часть и решаем как уравнение 7 степени x^7-3x^6-2x^5-3x^4+x^3=0

How I solve this: 1. Calculate sqrt(2), it's ~1.414. 2. Find the lack to get 2: 2-1.414=~0.586 3. Mutiply 0.586 two times to get a value we need to sqrt, it's ~0.3434. 4. Let's try: sqrt(2)+sqrt(0.3434), it's really close to 2. The answer is x=0.3434

Does not look like an olimpiad question at all, just some school equity for beginners

= 3363- 3362.999851 = 0.000148. (0.4 to the power 10 is very close to zero)

I can also use binomial expansion. I even made an identity for (a+b)¹⁰ still this works nice method though

Thats only one of the three solutions.

?=16^4×15=15×2^16=30×2^15

Pretty clever way of finding the exact value of x. Dr. Ajit Thakur (USA).

The solutions x = 1 and x = -6 are obvious (120 = 2 × 3 × 4 × 5). So just multiply it all out to get the equation in standard quartic form, divide the quartic by (x -1)(x + 6) = x² +5x - 6, and all that's left is a quadratic to solve.

(x+4)! = 5! --> x+4 = 5 --> x = 1

The answer is 2^16×15

(1+1)•(1+2)•(1+3)•(1+5)=2•3•4•5= 120=5! <=> x=1

Sqr(121)=+11 m^2=121 ==> m^2-121=0 ==> (m+11)*(m-11)=0 ==> m=11 or m=-11 sqr(x) is only >= 0

Si se hace el logaritmo complejo en el 5^x=-√(15) se podria hallar una solucion?

Very astucious change of variable. Love it.

That was good

এত মার প্যাঁচ করলেন,ছাত্র ছাত্রীরা না ঘাবড়ে যায়।

I just immediately saw that the four sets of brackets hold a series of numbers that are incremental. And since 2x3x4x5=120 I just assumed the answer was that X=1. So within 5 seconds I thought I had the answer. I really didn't consider there may be others possible. I really need to learn to be more pedantic with these kinds of problems.

120=5! = 5x4x3x2x1 solved

Helpful, informative Algebra solution

Awesome 👏 info as usual

It was satisfying to see how everything came to a neat conclusion though the path was long

(2)+2ab(4)=8(1)+1ab(2^2) (1^2) (ab ➖ 2ab+1).

The general solution for a+b can be written as a^2/(a-11). Now you can choose different options from there. The problem has infintely many solutions.

Thanks 🙏🏻 it will be more easy for beginners if music 🎵 is off and you explain

+/_4

La respuesta es V2

lg48/lg8.