
- 181
- 489 028
Prof. Georg Schildbach, University of Luebeck
Germany
เข้าร่วมเมื่อ 15 มี.ค. 2020
Vehicle Dynamics & Control - 23 Methods for vehicle motion planning
Vehicle Dynamics & Control - 23 Methods for vehicle motion planning
มุมมอง: 1 624
วีดีโอ
Vehicle Dynamics & Control - 22 Vehicle motion planning
มุมมอง 2Kปีที่แล้ว
Vehicle Dynamics & Control - 22 Vehicle motion planning
Technische Mechanik 1 - Aufgabenblatt 11 - Aufgabe 41
มุมมอง 1.1K3 ปีที่แล้ว
Technische Mechanik 1, Statik (Sommersemester 2020) Aufgabenblatt 11: Schnittgrößen am Balken mit Streckenlasten Lösung von Aufgabe 41 Link zum Aufgabenblatt 11: drive.google.com/file/d/1HtEr864w6otj0cJNMZtI1xXkvRRzG-GO/view?usp=sharing
Technische Mechanik 1 - Aufgabenblatt 10 - Aufgabe 37
มุมมอง 9963 ปีที่แล้ว
Technische Mechanik 1, Statik (Sommersemester 2020) Aufgabenblatt 10: Schnittgrößen am Balken Lösung von Aufgabe 37 Link zum Aufgabenblatt 10: drive.google.com/file/d/1zs_dq5VozzERqAyC-wk2BF33BAjfztwH/view?usp=sharing
Technische Mechanik 1 - 31 Beispiel 6: Schwerpunkt einer dreiecksförmigen Steckenlast
มุมมอง 7863 ปีที่แล้ว
Technische Mechanik 1, Statik (Sommersemester 2020) 6. Schwerpunkt Video 31 - Beispiel 6: Schwerpunkt einer dreiecksförmigen Steckenlast Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2018): Technische Mechanik Band 1 - Statik, 7. Auflage, Verlag Harri Deutsch. - Gross, Hauger, Schröder, Wall (2019): Technische Mechanik 1 - Statik, 14. Auflage, Springer Verlag. Gliederung: 1. Einl...
Vehicle Dynamics & Control - 00 Organization
มุมมอง 4.5K3 ปีที่แล้ว
Vehicle Dynamics & Control - 00 Organization
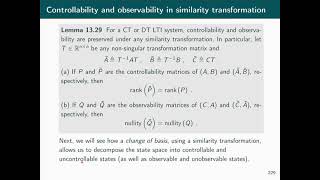
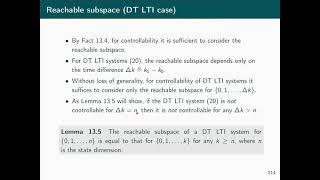
Linear System Theory - 13 Controllability and observability - part 2/2
มุมมอง 8603 ปีที่แล้ว
Linear System Theory Prof. Dr. Georg Schildbach, University of Lübeck Fall semester 2020/21 13. Controllability and observability - part 2/2 (controllable and observable canonical form, controllability and observability decomposition, stabilizability, detectability, Hautus test, Kalman decomposition) Link to lecture slides: drive.google.com/file/d/1HBTN28T7KPUWuJXvMmDX2bGadCZugpAL/view?usp=shar...
Linear System Theory - 13 Controllability and observability - part 1/2
มุมมอง 8343 ปีที่แล้ว
Linear System Theory Prof. Dr. Georg Schildbach, University of Lübeck Fall semester 2020/21 13. Controllability and observability - part 1/2 (controllability and observability of discrete-time and continuous-time systems) Link to lecture slides: drive.google.com/file/d/1HBTN28T7KPUWuJXvMmDX2bGadCZugpAL/view?usp=sharing (Copyright by Prof. Dr. Georg Schildbach) Course contents: 00. Organization ...
Technische Mechanik 2 - 43 Beispiel: Rutschende Masse auf Drehscheibe
มุมมอง 3743 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 43 - Beispiel: Rutschende Masse auf Drehscheibe Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Technische Mechanik 2 - 42 Arbeitssatz für den starren Körper
มุมมอง 7193 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 42 - Arbeitssatz für den starren Körper Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Technische Mechanik 2 - 41 Beispiel: Massenträgheitsmoment einer homogenen Kugel
มุมมอง 6873 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 41 - Beispiel: Massenträgheitsmoment einer homogenen Kugel Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Technische Mechanik 2 - 39 Massenträgheitsmoment
มุมมอง 6154 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 39 - Massenträgheitsmoment Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Technische Mechanik 2 - 38 Drallsatz für den starren Körper
มุมมอง 4314 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 38 - Drallsatz für den starren Körper Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Technische Mechanik 2 - 37 Dynamik des starren Körpers
มุมมอง 3634 ปีที่แล้ว
Technische Mechanik 2, Dynamik (Wintersemester 2020/21) 7. Dynamik des starren Körpers Video 37 - Dynamik des starren Körpers Die Vorlesung folgt den Lehrbüchern: - Hagedorn, Wallaschek (2016): Technische Mechanik Band 3 - Dynamik, 5. Auflage, Europa-Lehrmittel Verlag. - Gross, Hauger, Schröder, Wall (2015): Technische Mechanik 3 - Kinetik, 13. Auflage, Springer Verlag.
Linear System Theory - 12 Stability of state space models - part 1/2
มุมมอง 7754 ปีที่แล้ว
Linear System Theory Prof. Dr. Georg Schildbach, University of Lübeck Fall semester 2020/21 12. Stability of state space models - part 1/2 (Jordan canonical form, equilibrium, stability of discrete-time and continuous-time systems) Link to lecture slides: drive.google.com/file/d/1OiILCpLiI3FYDV13Kvts7ZcgXS2y1Kfu/view?usp=sharing (Copyright by Prof. Dr. Georg Schildbach) Course contents: 00. Org...
Linear System Theory - 12 Stability of state space models - part 2/2
มุมมอง 3934 ปีที่แล้ว
Linear System Theory - 12 Stability of state space models - part 2/2
Technische Mechanik 2 - Aufgabenblatt 12 - Tipps
มุมมอง 1984 ปีที่แล้ว
Technische Mechanik 2 - Aufgabenblatt 12 - Tipps
Linear System Theory - 11 Singular Value Decomposition and induced norms
มุมมอง 5104 ปีที่แล้ว
Linear System Theory - 11 Singular Value Decomposition and induced norms
Technische Mechanik - 36 Das Rückstoßprinzip
มุมมอง 5584 ปีที่แล้ว
Technische Mechanik - 36 Das Rückstoßprinzip
Technische Mechanik 2 - Aufgabenblatt 11 - Tipps
มุมมอง 2644 ปีที่แล้ว
Technische Mechanik 2 - Aufgabenblatt 11 - Tipps
Technische Mechanik 2 - Aufgabenblatt 11 - Aufgabe 50
มุมมอง 3434 ปีที่แล้ว
Technische Mechanik 2 - Aufgabenblatt 11 - Aufgabe 50
Technische Mechanik 2 - 35 Beispiel zum Drallsatz
มุมมอง 1.3K4 ปีที่แล้ว
Technische Mechanik 2 - 35 Beispiel zum Drallsatz
Technische Mechanik 2 - 34 Drallsatz für System von Massenpunkten
มุมมอง 5994 ปีที่แล้ว
Technische Mechanik 2 - 34 Drallsatz für System von Massenpunkten
Technische Mechanik 2 - 33 Arbeitssatz und Impulssatz für ein System von Massenpunkten
มุมมอง 5484 ปีที่แล้ว
Technische Mechanik 2 - 33 Arbeitssatz und Impulssatz für ein System von Massenpunkten
Technische Mechanik 2 - 32 Dynamik eines Systems von Massenpunkten
มุมมอง 5964 ปีที่แล้ว
Technische Mechanik 2 - 32 Dynamik eines Systems von Massenpunkten
Technische Mechanik 2 - Aufgabenblatt 10 - Aufgabe 42
มุมมอง 5194 ปีที่แล้ว
Technische Mechanik 2 - Aufgabenblatt 10 - Aufgabe 42
Technische Mechanik 2 - Aufgabenblatt 10 - Tipps
มุมมอง 2334 ปีที่แล้ว
Technische Mechanik 2 - Aufgabenblatt 10 - Tipps
Technische Mechanik 2 - 27 Arbeitssatz für den Massenpunkt
มุมมอง 1.1K4 ปีที่แล้ว
Technische Mechanik 2 - 27 Arbeitssatz für den Massenpunkt
Technische Mechanik 2 - 30 Schräger Stoss eines Massenpunkts auf einen glatten Boden
มุมมอง 3774 ปีที่แล้ว
Technische Mechanik 2 - 30 Schräger Stoss eines Massenpunkts auf einen glatten Boden
Technische Mechanik 2 - 31 Stoß zweier Massenpunkte
มุมมอง 4614 ปีที่แล้ว
Technische Mechanik 2 - 31 Stoß zweier Massenpunkte







Regarding proposition 10.5 (b): isn't the product of two hermitian matrices only hermitian if they commute
You are absolutely right. 10.5 (b) is incorrect and should be removed here. (I have already done so in my course slides.)
any book on this, it is difficult to read handwritten symbols
Prof. Is there a way to access these notes you have prepared throughout the course?
Legend
Sehr hilfreich, Danke
Professor, the part 18 is missing 😢
It is so important
Noch eine Frage um zu schauen ob ich es richtig verstehe. Zum Standbild bei 3:00. Das heißt, mit den beiden Regeln a) und b) ist es nicht möglich die drei Vektoren F_1, F_2 und F_3 GLEICHZEITIG zu einer Resultierenden zusammenzufassen. Der Grund ist, dass F_1, F_2 und F_3 keinen gemeinsamen Schnittpunkt haben. Man MUSS es so machen wie im Video, also erst zwei Kräfte zusammenfassen und dann die Resultierende mit der übrig gebliebenen Kraft so entlang der jeweiligen Wirkungslinie verschieben, sodass sie einen gemeinsamen Angriffspunkt haben um sie dann zusammenfassen. Sehe ich das richtig?
Korrekt.
Why using the refence point at the middle? Why not only consider the steering angle
hello and thank you for your Video. Is there a specific reference book for these descriptions?
Hallo Herr Schildbach, vielen Dank fürs Hochladen der tollen Videos. Gibt es für die anderen Aufgaben vom Aufgabenblatt 01 auch Musterlösungen? Auch wenn es nur die Lösungen ohne Rechenweg gibt, also nur die Lösungs-Zahlenwerte, wäre ich froh diese zu erhalten. Sie könnten die vlt in die Videobeschreibung packen. Oder als Kommentar unter dem Video.
Leider können wir aus ablauftechnischen Gründen der TM-Veranstaltungen an der Uni Lübeck die Lösungen nicht hier über TH-cam verfügbar machen. Ich bitte um Verständnis.
@@professorschildbach Das verstehe und akzeptiere ich natürlich. Wäre es aber möglich, dass Sie mir sagen ob meine Lösung zu Aufgabe 4 korrekt ist? a) Es gilt S_(1,y)=sinα∙S_1 S_(1,x)=cosα∙S_1 S_(2,x)=S_2∙sinβ S_(2,y)=-S_2∙cosβ Das negative Vorzeichen in S_(2,y), da S_(2,y) → (Vektorpfeile schreibe ich rechts daneben) in die negative y-Richtung zeigt. Somit gilt R_x=S_(1,x)+S_(2,x) und R_y=S_(1,y)+S_(2,y) ⇔R_x=S_1∙cosα+S_2∙sinβ und R_y=sinα∙S_1-S_2∙cosβ Die Wirkungslinie von R→ liegt in der Längsachse des Tankers, daher gilt: R → = (R_x , 0) , R_x >0 , 5 MN = R=√ ( R_x^2+ R_y^2 )=√( R_x^2 ) = |R_x | = R_x Somit erhalten wir durch umformen 5 MN=S_1∙cosα+S_2∙sinβ und 0=sinα∙S_1-S_2∙cosβ ⇔5 MN=S_1∙cosα+S_2∙sinβ und S_2=sinα/cosβ ∙S_1 ⇔5 MN=S_1∙cosα+sinα/cosβ ∙S_1∙sinβ und S_2=sinα/cosβ ∙S_1 ⇔5 MN=S_1∙(cosα+sinα/cosβ ∙sinβ ) und S_2=sinα/cosβ ∙S_1 ⇔(5 MN)/(cosα+sinα/cosβ ∙sinβ )=S_1 und S_2=sinα/cosβ ∙S_1 Die Gleichungen S_1=(5 MN)/(cosα+sinα/cosβ ∙sinβ ) S_2=sinα/cosβ ∙S_1 mit denen wir die Beträge der Seilkräfte ausrechnen können. b) Aus der vorherigen Aufgabe erhalten wir die Funktion: S_2 (β)=sinα/cosβ ∙S_1 Wir bilden die ersten beiden Ableitungen: S_2 ' (β)=(S_1 sin(α) sin(β))/cos^2(β) S_2 '' (β)=(S_1∙sin(α)∙(2∙sin^2(β)+cos^2(β) ))/cos^3(β) . Bestimmung von Extremstellen: S_2 ' (β)=0⇔(S_1 sin(α) sin(β))/cos^2(β) =0⇔S_1 sin(α) sin(β)=0⇔sin(β)=0⇔β=sin^(-1)(0)=0 Also ist β=0 ein Kandidat für eine Extremstelle. S_2 '' (0)=(S_1∙sin(α)∙(2∙sin^2(0)+cos^2(0) ))/cos^3(0) =(S_1∙sin(α)∙(0+1))/1=(S_1∙sin(α))/1=S_1∙sin(α) =S_1∙0.5>0 Da S_2 '' (0) >0 gilt, handelt es sich bei β=0 um ein lokales Minimum.
Eine Frage zu 3:22, also, dass wir uns den Körper als unendlich ausgedehnt vorstellen sollen. Wenn ein Schnittpunkt von mehreren Kräften außerhalb des starren Körpers liegt und ich diese Kräfte zu einer Resultierenden zusammenfasse, dann kann ich ja diese Resultierende wieder entlang ihrer Wirkungslinie verschieben. Nun kann ich doch die Resultierende so entlang ihrer Wirkungslinie verschieben, dass sie wieder innerhalb des Körpers liegt, oder? Damit braucht ich diese Vorstellung von einem unendlich ausgedehnten Körper doch gar nicht 🤔
Wenn das möglich ist, kann man das machen. Aber der Schnittpunkt zweier Wirkungslinien liegt vielleicht erstmal außerhalb des Körpers. Außerdem kann es sein, dass die Wirkungslinie gar nicht durch den Körper geht.
@@professorschildbach Danke für Ihre Antwort!
Hallo, erstmal vielen Dank für diese tollen Videos. Ich hätte eine Frage: Betrachten wir das Buch auf dem Tisch in Ihrem Video th-cam.com/video/-ERlA3pIKgI/w-d-xo.html bei 7:00. Was sind hier die Actio-reactio-Paare? Ich würde denken, die Gravitationskraft die die Erde auf das Buch ausübt, welche im Video mit G bezeichnet wird und im Schwerpunkt des Buches angreift und die Gravitationskraft die das Buch auf die Erde ausübt und im Schwerpunkt der Erde angreift. Diese beiden Kräfte sind ein Actio reactio Paar. Dann die Kraft die der Tisch auf das Buch ausübt und im verlinkten Video mit F_Tisch bezeichnet wird und eine Kraft F_Buch die das Buch auf den Tisch ausübt. F_Tisch und F_Buch sind ein Actio reactio Paar. Aber ist F_Buch nicht identisch zu G ? Ich würde denken ja, und das würde dann bedeuten, dass eine Kraft in zwei Actio-reactio-Paaren auftaucht. Oder sehe ich hier was falsch?
Ahh ich glaube ich hab's. F_Buch ist nicht identisch zu G denn G greift im Schwerpunkt vom Buch an wohingegen F_Buch am Tisch angreift. Diese beiden Kraftvektoren sind also verschieden. Aber vom Betrag und von der Richtung her, sind G und F_Buch gleich und ich denke ich kann das auch mathematisch zeigen: Wir betrachten, dass Buch und wissen, dass F_res → = G → + F_Tisch → und F_res → =m∙a → und a → = 0 → gilt (ich schreibe die Vektorpfeile rechts daneben, weil ich sie hier nicht über dem Buchstaben schreiben kann). Durch umformen F_res → = G → + F_Tisch → und F_res → =m∙a → und a → = 0 → ⇔ F_res → = G → + F_Tisch → und G → + F_Tisch → = 0 → und a → = 0 → ⇔ F_res → = G → + F_Tisch → und G → = -F_Tisch → und a → = 0 → erhalten wir G → = -F_Tisch → . Weiterhin wissen wir, dass F_Tisch → = - F_Buch → gilt, denn F_Tisch → und F_Buch → sind ein Actio-reactio-Paar. Durch umformen F_Tisch → = - F_Buch → ⇔ G → = -F_Tisch → = F_Buch → erhalten wir schlussendlich G → = F_Buch →. Wären die Vektoren G → und F_Buch → freie Vektoren, dann wären sie laut dieser Rechnung identisch. Da sie aber beide gebudene Vektoren sind und verschiedene Angriffspunkte haben, handelt es sich bei ihnen um verschiedene Vektoren aber mit derselben Richtung und demselben Betrag. Passt das alles so?
@@back2back135 Ja, das ist alles so richtig! 👍
@@professorschildbach ich danke Ihnen
Vielen Dank für das Video
5:50 Was stellt dieses zweite Integral dar? Warum macht die Gesamtformel Sinn?
Thank you for these videos! I am a game developer working independently on my own vehicle systems, these videos are really useful and good to watch in-between sessions of work
Hahahaha I am the exact opposite. I am an automotive engineering student who develops video games in my free time :D funny to see the same case as me in an opposite manner
what about truck with trailer model?
Thank you Professor, very clear. I have a question with the last step in the derivation. Unfortunately my high school trig is lacking. So to anyone reading this, if you could enlighten me it would be most appreciated (exam's next Tuesday :/). How do you get from (1/lr+lf)*tan(d)*cos(B) ---to---> B = atan[(lr/lf+lr)*tan(d)] ?
You get there from tan(B)=lr/(lf+lr)*tan(d). Good luck on the exam!
@@professorschildbach Ah ok, so you just used the tan(B) which = lr/R(||) and then substituted in for R(||). The switch from cos to tan threw me for a second. But because the angle B can be expressed in any of the following ways sin(B)=lr/R, or cos(B)= R(||)/R, or tan(B)=lr/R(||) it's all the same. And you needed to find R first that's why used cos(B) instead of going straight to tan. Thanks for getting back to me
I'm a math student and somewhat new to this engineering content. At around 6:40, would it be correct to say that the path frame will be the usual tangent, normal, binormal vector frame one would get from vector calculus? The d-axis being the tangent vector, e-axis being the normal vector, and n being the binormal vector?
Yes, that would be correct.
I am actually studying in another city, and we do not have this kind of high quality course on Vehicle Dynamics, but I need some knowledge about it for a big project. Therefore, I am really glad that you are sharing this course.
clear and easy Thank u
Prof. can I please have the notes? They are amazing.
FWD cars understeer because they have more weight over the front, but lift-off oversteer occurs because the car's weight transfers forward.
Thank you for these courses, really well explained
I think if the gear ratio greater than 1, means the speed of wheels slower than the shaft; if less than 1, means the speed of wheels faster than the shaft. However, that is different what professor said ih the lecture
Hi Prof, excellent explanation! Thank you so much! However, why the anti dive is only analyzed on the front wheel not the rear? What about using the rear-axle only hand brake to brake the car? I did a similar calculation using your anti squat example with rear wheel drive model and got the rear axle weight loss. Is that always the case? A driving instructor once told me using rear brake will "tuck" the car in, I guess he meant squat. But that will by no means happen, right?
Thank you Prof Schildbach for this video. I have a question that we know the kinematic of a bicycle can be written as a nonlinear system like \dot x = f(x,u) and how to check controllability of this system?
That was great and I agree with the previous comment. And I like the notion of adjusting the pivot points for a more enjoyable driving experience for a standard vehicle. So, if I am a little below and above the pivot points respectively, am still OK unless I am doing a dragster. And that would be its own driving experience LOL.
Professor, may I ask when to use rear wheel reference and front wheel reference since mostly we use center point reference. Thanks
It is difficult to give a general answer. The the rear axle is typically used as a reference point for low-speed applications (like parking). The CoG is often used as a reference point for high-speed applications. The front wheel is rarely used, in my experience. However, it is used, for example, as the reference point for the Stanley Controller.
Sehr gutes Video, dankeschön.
Danke fürs Hochladen.
Eine Frage: Im Buch "Technische Mechanik 1" von Gross steht "Die Richtung der Kraft können wir durch ihre Wirkungslinie und den Richtungssinn auf ihr beschreiben." Das heißt, Richtung = Wirkungslinie + Richtungssinn . Die Vektoren bei 4:24 haben dieselbe Wirkungslinie aber einen verschiedenen Richtungssinn deshalb ist die Richtung der beiden Vektoren verschieden. Oder sehe ich da was falsch?🤔🤔 Es scheint, dass bei Ihnen mit "Richtung" das gemeint ist was in Gross mit "Wirkungslinie" bezeichnet wird.
Danke für den Hinweis. Wir verwenden hier die Terminologie aus dem Buch "Technische Mechanik 1" von Hagedorn und Wallaschek. In dem von Ihnen genannten Buch wird eine leicht andere Terminologie verwendet. Wichtig ist nur, dass die Terminologie konsistent ist, damit die Bedeutung immer klar und eindeutig ist. Und Sie sollten natürlich die Terminologie aus Ihrem Kurs verwenden.
@@professorschildbach Danke für die Antwort und für das Hochladen der Videos.
Danke fürs Hochladen!!!
What is my cars front n rear trackwidth is different?..how will I calculate the roll angle..and what will be the value of "b"?
Wo bleibt Tm3 😊?
How would you work out a pivot point for a trailing arm suspension?
this is the best explanation of anti-dive and anti-squat i've seen, just because it's in terms of moments and not just geometry (and trust).
I have never seen this degree of clarity in my life, not in kinematics at least. I did not want to comment again so as not to annoy, but I had to one more time. Once again Thank you so much.
Thank you so much for such a clear presentation of fundamentals. These lectures are a gem.
could you recommend some relative textbooks, which contain more details like explaining the induction of the equations in your video? thanks a lot.
It appears to me that, for a 3-dimensional rigid body, the instantaneous centre of rotation is not a single unique point, but rather all points along some line.
Yes, the instantaneous center of rotation only exists for planar (or quasi-planar) motion.
I have a question regarding a research paper I found dealing with vehicle dynamics in oversteer. It deals with elliptical tire models and load transfer, and I can’t understand some of the substitutions the writer makes into the equations of motion. I already sent you an email named “Vehicle dynamics question” through your university email address. It would be very helpful if you can reply to it. I really enjoy your videos
I am glad you like the subject and you enjoy my videos. Please understand that I lack the time to support individual projects or explain details in papers which are not covered in my lectures. Best of luck with your project!
Sir, I have a question. Why would you make an assumption of the small steering angle and why would sine and tanjent of it be delta, not 0?
Small steering angle assumption: We are engineers. It's a choice to make this assumption. You make in cases where you deem the assumption of a "small" steering angle as justified (e.g., on highways maybe?). Small angle approximation: These are the first order Taylor approximations of the sine and tangent functions.
Really good lecture. Can I translate it into Chinese and repost it on bilibili? I'll mark your name and this youtube address there
Yes, you can. Thanks for asking.
@@professorschildbach Thank you sir.
7:45 should be 1 rad/s = 30/pi rpm
Right. Thank you for your this correction.
genio
Bitte warum gibt es ein Minus beim omegazwei ( W2)
Über welche Stelle im Video reden wir hier?
Wow this is a great explanation!
what a master class! I can not believe this is free! but x1.75 is the way to go xD